Chapter 3: Data Handling

INTRODUCTION 
    In this chapter we will learn
    1.    Various forms of data        
    2.    Organization of data
    3.    Useful forms of data representation.
    Data: Information presented in the form of numerical figures. For example:
    “Rajesh has 15 books” is a data whereas nearly saying that         
    “Rajesh has large number of  books” is not a data.
    

•          Raw Data: Data as obtained from the source, generally in unarranged form.

Chapter 3

Data handling

Arithmetic mean

Data handling may be as simple as drawing on a sheet of paper or the completion of entry forms on a computer screen. Data is a collection of numbers gathered to give some information
For eg the marks of students in the class or the amount of various goods shopped from the market.

TYPES OF DATA
    There are two types of data:
    (a)       Primary data: Data collected by a person directly (by himself) is called primary data.
    (b)       Secondary data : Data  which are already collected by a person or a society and these may be in published or unpublished sources are known as secondary data. These data should be carefully used. 
    For Example
    (i)     Data published by the state or central governments.
    (ii)     Reports published by international organisations like U.N.O.

    The data collected by someone for himself and used by someone else is called secondary data.
    Observation: Each numerical figure in the data is called an observation.
    For example, the marks obtained by 30 students in mathematics (out of 40) are:
        30, 25, 18, 25, 39, 18, 25, 30, 28, 17,
        15, 30, 30, 35, 36, 36, 35, 30, 25, 20,                   
        25, 28, 18, 17, 16, 30, 36, 35, 27, 28.               

1.    Array
    Sequentailly arranged data either in ascending or descending order is called an array.
    This is a raw data and the marks obtained by each student is an observation. Thus, there are 
    30 observations in this raw data.
    We arrange the above marks in ascending order as below:
        15,  16,  17,  17,  18,  18,  18, 20, 25, 25,
        25, 25, 25, 27, 28, 28, 28, 30, 30, 30,
        30, 30, 30, 35, 35, 35, 36, 36, 36, 39

2.    Range: The difference between the highest and lowest observation in a given data is called its range.

    Illustration 1
        Find the   range for the following data:
        45, 47, 45, 86, 62, 80, 48, 41, 31, 51, 99, 80, 78. 
    Solution   
        The smallest observation = 31 The largest observation = 99
        Range = 99 – 31 = 68

3.    Frequency: Number of times occurrence of a particular information (observation) is called the frequency of that information (observation).
    (i)     Ungrouped Frequency Distribution :    If  the data is large and not classified in groups then for there representation we use tally marks according to there frequency this distribution is known as ungrouped frequency distribution. 
    (ii)      Grouped Frequency Distribution :  When the list of observations is long, the data is usually organized into groups called class intervals and the data so obtained is called a grouped data.

•         Lower Limit and Upper Limit : The lower value of a class interval is called its lower limit and the upper value of class interval is called its upper limit.
•         The size or the Width of the Class Interval : The difference between the upper and the lower class limits is called the size or width of the class interval. 

                   For example :
        The size of the class intervals = Upper Limit – Lower Limit

•         Class Marks :  The mid value of a class interval is called its class mark. It is obtained by adding its upper and lower limit and dividing by 2.

4.    Tally Marks: Figurative representation of frequency using vertical lines. The use of tally marks can be understood from the undetermined illustration.

    Illustration 2
        Given below is the data showing the number of cars in 25 families of a locality.
        3, 1, 3, 2, 2, 2, 0, 3, 4, 2, 1, 3, 2, 4, 1, 2, 2, 3, 1, 3, 4, 0, 1, 2, 4, 
        Arrange the data in ascending order and prepare the frequency table.
    Solution    
        Arranging the data in ascending order we get
        0, 0, 1, 1, 1, 1, 1, 2, 2, 2, 2, 2, 2, 2, 2, 3, 3, 3, 3, 3, 3, 4, 4, 4, 4
        Table for the same is represented as below. We have introduced a column tally marks which represents the frequency of a number.

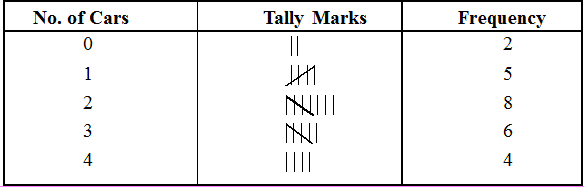
Illustration 3
        In an examination the marks obtained by 20 students of class VII are given below :
        14, 2, 7, 12, 15, 16, 22, 13, 24, 2, 26, 5, 29, 18, 31, 36, 38, 1, 47, 4.  
        Make a frequency distribution table of same class size and one such class being 0 – 10.
    Solution

Mode

The mode of a set of observations is the observation that occurs most often.
When the number of observations is large, observing them together is not easy. In case of large data, tabulation can be done by putting tally marks and finding the frequency. The observation with the highest frequency is the mode of the data.

Median

Median refers to the value which lies in the middle of the data when data is arranged in an increasing or decreasing order. Half of the observations lie above the median and the other half below it.
To calculate the median, the data is first arranged in an ascending or descending order. Then the middle value is observed.
For eg; 2, 4, 6, 8, 9
6 is the median in this case since it lies in the middle. There are two observations above and below 6.
In case of odd number of observation, the value of median is one of the observations in the existing data.
In case of odd number of observation, the value of median is one of the observations in the existing data.
In case of even number of observations, the value of median is not one of the observations in the existing data.

Bar graph

A bar graph is a representation of numbers using bars of uniform width and the lengths of the bars depend upon the frequency and the scale you have chosen.
It is a method of representing data visually. In the case of bar graph or bar diagram, Bars of uniform width are drawn horizontally or vertically with equal spacing between them and then the length of each bar represents the given number.

Drawing a Bar Graph

To draw a bar graph, first of all draw a horizontal line and a vertical line.
On the horizontal line we will write the name of students and on vertical line we will write numerals which show what data is being represented.
Same data can also be represented by interchanging the items on horizontal and vertical axis.
It is important to take bars of same width keeping uniform gap between them. Next, a scale is chosen if needed.
The scale varies according to the data given. One block is equivalent to ten units.

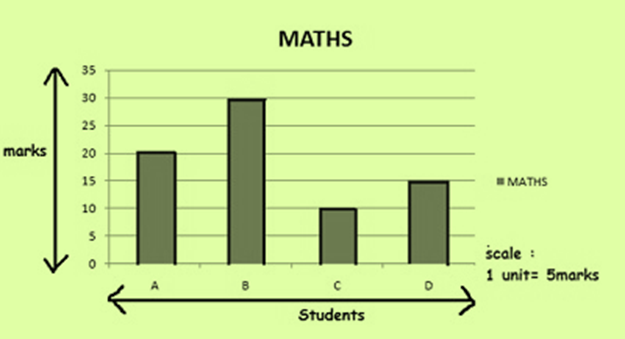
Example 1: Draw simple bar graph .Let there be 4 students A,B,C and D. The total marks Maths test is 40.

Answer:-

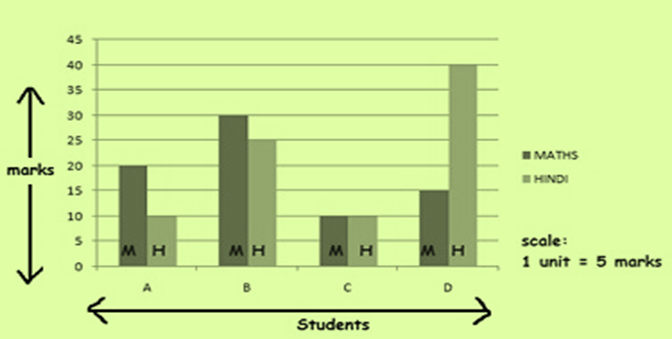
Double Bar Graph

A simple bar graph represents one set of data. For e.g score of students in a Maths.
A bar graph is drawn in a similar way to simple bar graph.
A double graph represents two sets of data in one graph.For eg score of students in Maths and Hindi.

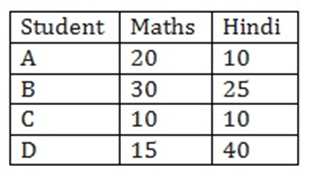
Example 2 :  Let there be 4 students A,B,C and D. The total marks for both hindi and Maths test is 40.double bar graphs can be obtained as:

Answer:-

MEAN OF UNGROUPED DATA
    Mean is statistics is same as the average in arithmetic.

Illustration 4
        Find the mean of the following data: 4, 2, 3, 9, 6, 5, 1.
    Solution
        Sum of given numbers (observations) = 30
        number of given numbers (observations) = 7    

Illustration 5
        Following are the ages (in years) of 10 teachers in a school: 42, 28, 32, 41, 33, 54, 27, 23, 34, 37. 
        (a)     What is the age of the oldest teacher and that of the youngest teacher ?
        (b)     What is the range ?
        (c)     What is the mean age of the teachers ?
        (d)     What will be the new mean age if a new teacher aged 34 years is employed?  

Solution 
        (a)     Clearly the age of the oldest teacher in the school is 54 years and that of the youngest teacher is 23 years.
        (b)     The range  = 54 - 23 or 31 years   

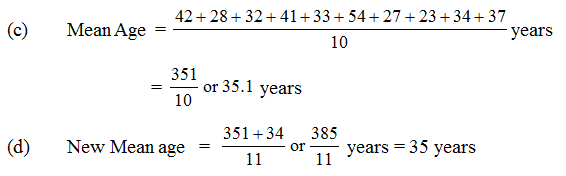
     Illustration 6
        Mean attendance of a class from Monday to Saturday was 33. If the mean attendance of Monday, Tuesday and Wednesday was 32 and that of Wednesday, Thursday, Friday and Saturday was 34, find the attendance on Wednesday.
    Solution 
        Mean attendance from Monday to Saturday = 33
        Total attendance from Monday to Saturday = 33 x 6 = 198                      ...(i)
        Mean attendance of Monday, Tuesday, Wednesday = 32     
        Total attendance of Monday, Tuesday, Wednesday = 32 x 3 = 96        ...(ii) 
        Mean attendance of Wednesday, Thursday, Friday, Saturday = 34 
        Total attendance of Wednesday, Thursday, Friday, Saturday
                        = 34 × 4 = 136                                                  ...(iii)
        From (ii) and {iii), 
        we get Total attendance from Monday to Saturday + Wednesday
                        = 96 + 136 = 232                                  ...(iv)
        From (i) and (iv), we get 
        Attendance on Wednesday = 232 – 198 = 34

Chance and probability

There are situations in our life, that are certain to happen, some that are impossible and some that may or may not happen. The situation that may or may not happen has a chance of happening.

Probability is the chance of occurrence of an event. It tells us about the likelihood of the occurrence or non occurrence of an event. Events that have many possibilities can have probability between 0 and 1.

Mode of Ungrouped Data
    Mode: The value which occurs most is called mode. In other words the value of the observation which has maximum frequency in a data is called mode.

    Illustration 10
        The marks obtained by 10 students are as follows : 
        5, 6, 3, 7, 4, 6, 9, 2, 1, 6
        Find the mode of the marks.
    Solution
        Arranging the data in ascending order 
        We get 1, 2, 3, 4, 5, 6, 6, 6, 7, 9
        We can see that 6 occurs most of the time, hence 6 is the mode.

    Illustration 11
        Find the mode of the following data: 
        (i)     2, 2, 3, 3, 4, 4, 4, 5, 6, 6, 7
        (ii)     31, 35, 36, 33, 32, 35, 36, 31, 34, 33, 36, 34, 32, 32, 36, 34. 
    Solution    
        (i)     4 occurs the maximum number of times, i.e., 3
        ∴    Mode = 4 
        (ii)     Arranging data in the ascending order, we get
            31, 31, 32, 32, 32, 33, 33, 34, 34, 34, 35, 35, 36, 36, 36, 36. 
            Here 36 occurs the maximum number of times, i.e., 4

    Illustration 12
        Find the mode from the following data:

Solution   
        Here the maximum frequency is 7. 
        The marks obtained against 7 is 43 
        Hence,    Mode = 43 marks

MEDIAN OF UNGROUPED DATA
1.    MEDIAN
    The median is the value which is strictly in the middle of the list of the observations when written in order of size, i.e., in ascending or descending order. It is also a measure of central tendency.
    (i)    When the number of observations is odd, then there will be only one mid term and this term is the median. 
           n = number of terms
           If n = odd

(ii)    When the number of observations is even, then there will be two middle terms. The average of these middle terms will be the median of the data.
        If n = even

Illustration 8
        Find the median of:
        (i) 5, 9, 6, 4, 7, 2, 8                 (ii) 2, 5, 3, 2, 4, 5, 2, 4, 6, 8, 7, 9, 7.

Illustration 9
        Find the median of the following data:
             11, 10, 12, 9, 8, 16, 15, 14    

Graphical Representation of Data
    A Bar graph is a graphical representation of the numerical data by a number of bars (rectangles) of uniform with erected horizontally or vertically with equal spacing between them. 
    Graphical representation of numericals data in the form of bars with fixed (equal) width and varying height.
    Method to draw Bar Graphs : 
    Step 1 : Draw a horizontal line OX also called as x-axis and a vertical line OY also called as y-axis.
    Step 2 : Mark points at equal intervals along x-axis. Write the names of the data whose values are to be plotted.
    Step 3 : After choosing a suitable scale, determine the heights of the values of the respective data names.
    Step 4: Mark off these heights parallel to the y-axis from the points taken in step 2.
    Step 5 : On the x-axis draw bars of equal width for the heights marked in step 4. The bars should be centered on the points marked on the x-axis. These bars represent the given numerical data.

Illustration 13
        In a particular section of Class VIII, 40 students were asked about the months of their birth and the following graph was prepared for the data so obtained :

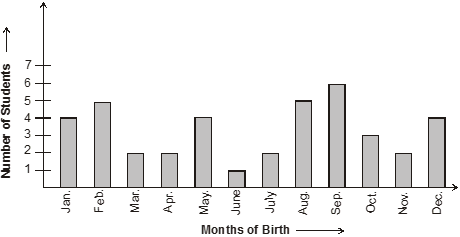
        (i)     How many students were born in the month of November ?
        (ii)     In which month were the maximum number of students born ?
        (iii)     In which month were the minimum number of students born ?
Solution
        Note that the variable here is the ‘month of birth’, and the value of the variable is the ‘Number of students born’.
        (i)     2 students were born in the month of November.
        (ii)     The maximum number of students were born in the month of September.
        (iii)     The minimum number of students were born in the month of June.