1. Perimeter of Rectangle and Regular Shapes
- Books Name
- CBSE Mathematics for Class 6
- Publication
- Carrier Point
- Course
- CBSE Class 6
- Subject
- Mathmatics
Notes adding
1. Perimeter of Rectangle and Regular Shapes
- Books Name
- CBSE Class 6 Mathematics Book
- Publication
- Param Publication
- Course
- CBSE Class 6
- Subject
- Mathmatics
Introduction
In this chapter we study the regions and boundrys of sum plain figures. We need some measures to compare them. We look into these now.
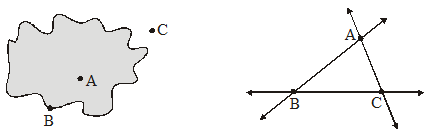
1. Perimeter of Rectangle and Regular Shapes
- Books Name
- Class 6 Mathematics Book
- Publication
- ReginaTagebücher
- Course
- CBSE Class 6
- Subject
- Mathmatics
Chapter 10
Mensuration
Perimeter of Rectangle and Regular Shapes
Introduction to perimeter,
Any shape that lies on a flat exterior and has only two extent i.e. length and breadth are called 2- D (two- dimensional) shape.
Polygons are closed numbers which are bounded by a chain of line parts, for illustration, triangles, squares, and squares. Perimeter (peri around; meter measure) of a polygon is the distance or direct measure of these bounded line parts. In other words, it is the length of its boundaries. Its unit is centimetre (cm) or meter (m).
Perimeter of a rectangle,
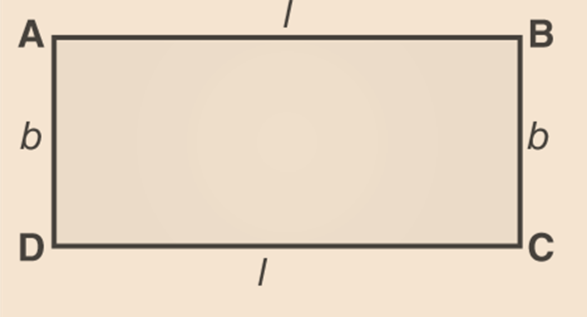
Rectangle is a four- sided polygon having two range i.e. length and breadth.
Perimeter of a cube = sum of four sides
= AB + BC+ CD+ AD
= length + breadth +length+ breadth = 2 length +2 breadth
Perimeter of a rectangle = 2 × (length+ breadth)
Perimeter of a square

Square is also a polygon where all its sides are the same.
The perimeter of a square = sum of all four sides
= a + a + a+ a
= 4a
The perimeter of a square = 4 × side
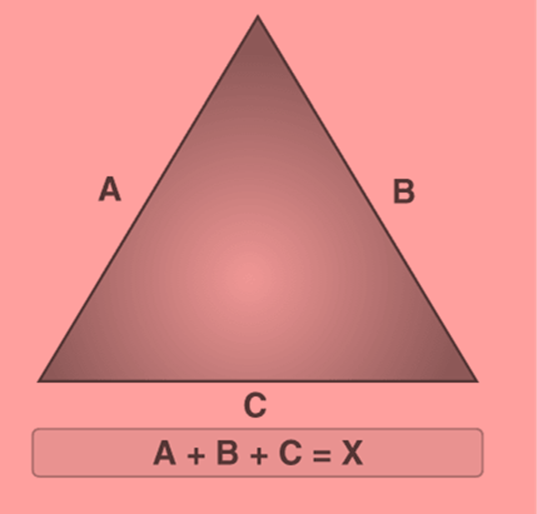
The sum of the lengths of the sides is the perimeter of any polygon. In the case of a triangle,
Perimeter = Sum of the three sides
Always include units in the final answer. If the sides of the triangle are measured in centimetres, then the final answer should also be in centimetres.
Perimeter of a triangle
The formula for the perimeter of a closed shape figure is usually equal to the length of the outer line of the figure. Therefore, in the case of a triangle, the perimeter will be the sum of all the three sides. If a triangle has three sides a, b and c, then,
Perimeter, P = a + b +c
Perimeter of an Isosceles, Equilateral and Scalene Triangle
Below table helps us to understand how to find the perimeter of different triangles- Equilateral triangle, Isosceles triangle and Scalene triangle.
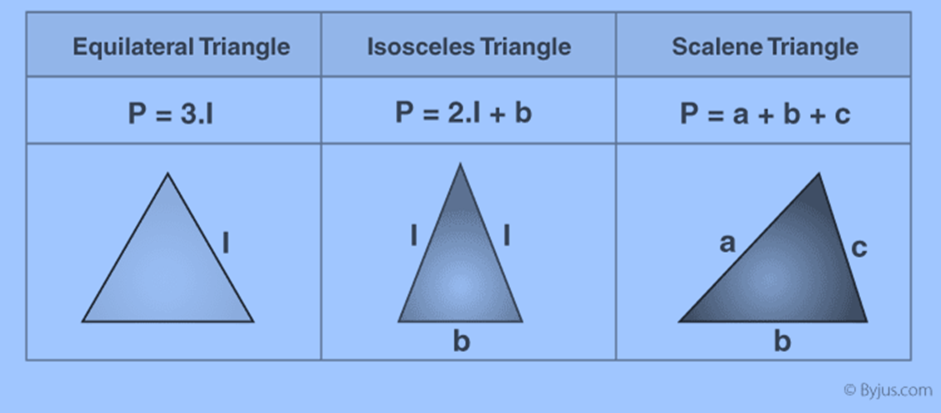
Perimeter of regular shapes
Perimeter of a parallelogram with Base, Height and an Angle
The perimeter of the parallelogram with base and height is given using the property of the parallelogram. If “b” is the base of the parallelogram and “h” is the height of the parallelogram, then the formula is given as follows:
According to the property of the parallelogram, the opposite sides are parallel to each other, and the parallelogram perimeter is defined as two times of the base and height.
Thus, the formula for the perimeter of a parallelogram is
P = 2 (b +h/cos θ)
where θ is the angle BAE, formed between the height and side of the parallelogram, i.e. AE and AB
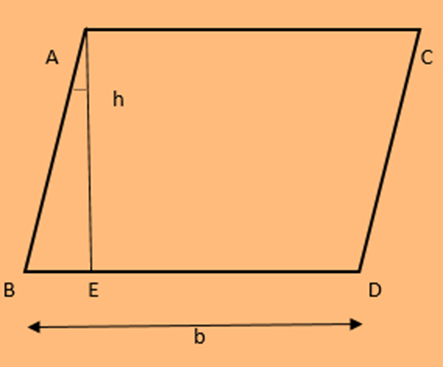
2. Area of Rectangle and Regular Shapes
- Books Name
- CBSE Class 6 Mathematics Book
- Publication
- Param Publication
- Course
- CBSE Class 6
- Subject
- Mathmatics
Perimeter
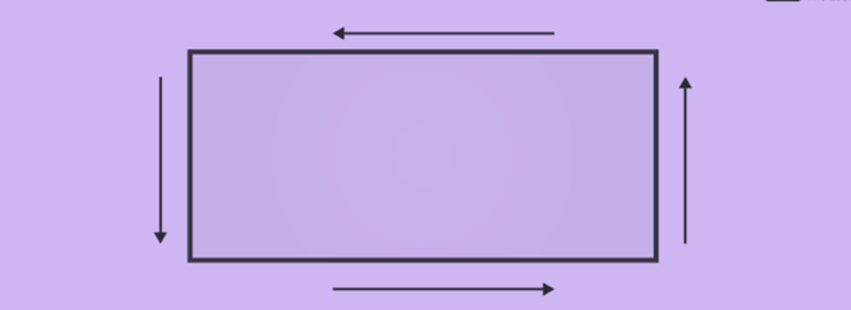
The perimeter of a closed figure is the length of the boundary of the figure.
The idea of perimeter is widely used in our daily life.
- A farmer who wants to fence his field.
- An engineer who plans to build a compound wall on all sides of a house.
- A person preparing a track to conduct sports.
All these people use the idea of ‘perimeter’.
1. Perimeter of Rectangle: The perimeter of a rectangle is the sum of all its sides. The opposite sides of rectangle are equal. If one side is l unit and the other side is b unit, then
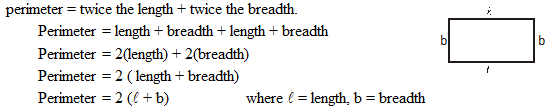
2 . Perimeter of a square: All four sides of a square are equal. If one side of a square measures 'a' unit, we can say that both the length and the breadth are a unit each.
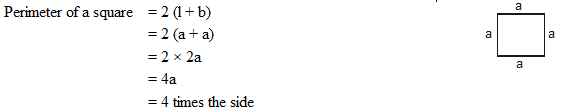
The perimeter of a square is equal to four times the length of its sides.
3. Perimeter of a triangle :
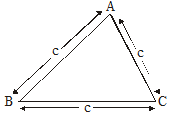
Perimeter = AB + BC + CA = a + b + c
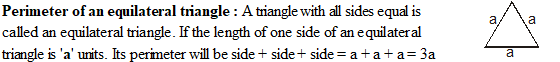
Note: In general, if the sides of a polygon are equal, that is, if it is a regular polygon, its perimeter will be the product of the length of its side with the number of sides.
The perimeter of a regular pentagon = 5a units
The perimeter of a regular hexagon = 6a units
The perimeter of a regular octagon = 8a units
where a is the length of one side.
Ex.1 Pinky runs around a square field of side 75 m, Bob runs around a rectangular field with a length of 160 m and breadth of 105 m. Who covers more distance and by how much?
Sol.: Distance covered by Pinky in one round = Perimeter of the square
= 4 × length of a side
= 4 × 75 m = 300 m
Distance covered by Bob in one round = Perimeter of the rectangle
= 2 × (length + breadth)
= 2 × (160 m + 105 m)
= 2 × 265 m = 530 m
Difference in the distance covered = 530 m – 300 m = 230 m.
Therefore, Bob covers more distance by 230 m.
Ex.2 Find the perimeter of a regular pentagon with each side measuring 3 cm.
Sol.: This regular closed figure has 5 sides, each with a length of 3 cm.
Thus, we get
Perimeter of the regular pentagon = 5 × 3 cm = 15 cm
Ex.3 A piece of string is 48 cm long. What will be the lenght of each side if the string is used to form:
(i) a square (ii) an equilateral triangle (iii) an regular octagon .
Sol. (i) one side of the square = Perimeter ¸ 4 = 48 ¸ 4 = 12 cm
(ii) one side of the equilateral triangle = Perimeter ¸ 3 = 48 ¸ 3 = 16 cm
(iii) one side of regular octagon = Perimeter ¸ 8 = 48 ¸ 8 = 6 cm
2. Area of Rectangle and Regular Shapes
- Books Name
- Class 6 Mathematics Book
- Publication
- ReginaTagebücher
- Course
- CBSE Class 6
- Subject
- Mathmatics
Area of Rectangle and Regular Shapes
Introduction to area,
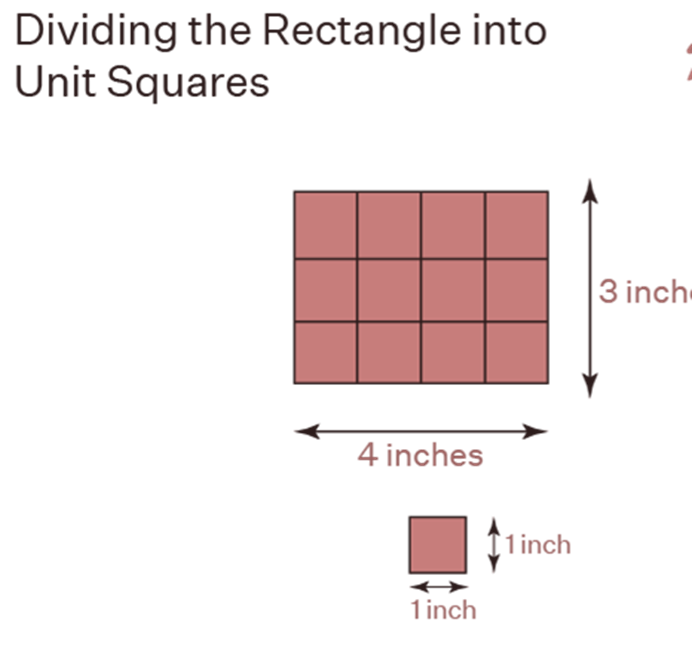
The area of any shape is the number of unit squares that can fit in to it.The area can be defined as the amount of space covered by a flat exterior of a particular shape. It is measured in terms of the" number of" square units (square centimetres, square height, square bases, etc.)
Area of the rectangle,
Description Area of rectangle is the number of unit squares within the boundary of the rectangle. Alternately, the space caught up within the border of a rectangle is called the area of the rectangle. One good illustration of a rectangle shape is the lines of unit length in your house. You can freely figure out how important space the bottom occupies by counting the number of penstocks. This will also help you determine the area of the cubic floor.region involved by a rectangle within its four sides or boundaries.
A = lb
How to Calculate the Area of a Rectangle
Follow the way below to find the area
Step 1 Note the range of length and breadth from the given data
Step 2 Multiply length and breadth values
Step 3 Write the answer in square units

Area of the square,
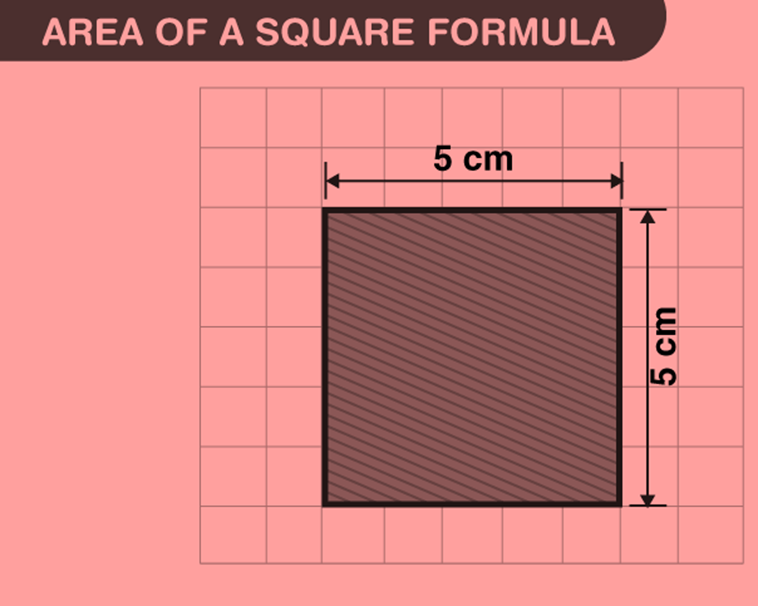
Area of a square is defined as the number of square units required to fill a square. In general, the area is defined as the region involved inside the boundary of a flat object or 2d figure. The dimension is done in square units with the standard unit being square measures (m2).
The area of square formula used for calculating the region involved, let us try using graph paper. You are needed to find the area of a side 5 cm. Using this dimension, draw a square on a graph paper having 1 cm × 1 cm squares. The square covers 25 complete squares.
Therefore, the area of the square is 25 square cm, which can be written as 5 cm × 5 cm, that is, side × side.
From the above discussion, it can be inferred that the formula can give the area of a square is
Area of a Square = Side × Side
Thus, the area of square = Side2 square units
And the perimeter of a square = 4 × side units
To find the area by counting the squares in the graph
The area of 1 full square can be taken as 1sq. unit.
Still, then the area can be considered as 1sq, if the region covers another than half the square. Unit.
Still, besides that region is neglected, if the region covers smaller than half the square.
Yet, also, the area is taken as 12sq, if the region covers exactly half the square. Unit.
These conventions are required to have in mind while chancing the area of the shape from the graph.
Sample
Find the area of the given shape by counting the squares.
Answer

Let us count the number of squares.
Number of completely- filled squares = 6
Area of completely- filled squares = 6 × 1 = 6sq. cm
Number of half- filled squares = 2
Area of half- filled squares = 2 × 12 = 1sq. cm
Number of further than half- filled squares = 4
Area of other than half- filled squares = 4 × 1 = 4sq. cm
Number of lesser than half- filled squares = 2
Area of smaller than half- filled squares can be neglected.
Total area covered by the given shape = (6 1 4) cm = 11sq. cm
Therefore, the total area covered by the given shape is 11sq. cm.
Area
- Books Name
- CBSE Class 6 Mathematics Book
- Publication
- Param Publication
- Course
- CBSE Class 6
- Subject
- Mathmatics
Area
The amount of surface of the plane covered by a closed figure is called its area. For every closed figure, there are two regions.

The term 'area' refers to the measure of the total interior region.
Closed figures given below. They are occuping some region it is difficult to tell which figure occupies more region. It is difficult to make out unless we measure the area.
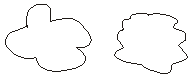
In order to calculate which closed figure having larger area we place them on a squared paper or graph paper where every square measures 1 cm × 1 cm. Make an outline of the figure.
Look at the square enclosed by the figure. Some of them are completely enclosed some half, some less than half and some more than half.
The area is the number of centimeter squares that are needed to cover it.
But there is a small problem that the square do not always fit exactly into the area we measure. We get over this difficulty by adopting a convention.
- The area of one full square is taken as 1 sq unit. If it is a centimetre square sheet , then area of one full area will be 1 sq. cm.
- Ignore portions of the area that are less than half a square.
- If more than half of a square is in a region, just count it as one square.
- If exactly half the square is counted , take its area as sq unit.
Such a convention gives a fair estimate of the desired area.
Ex.1 Find the area of the shape shown in the figure.
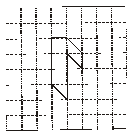
Sol. This figure is made up of line segments moreover, it is covered by full squares and half squares only. This makes our job simple.
(i) fully filled squares = 3
(ii) half filled squares = 3
Area covered by full squares = 3 × 1 sq units = 3 sq units
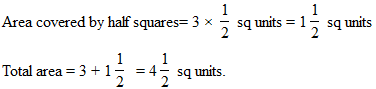
1. Area of rectangle: For rectangle having length l unit and breadth b units.
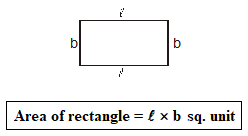
2. Area of square: For square having side a unit.

3. Area of a triangle :
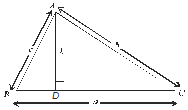
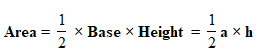
Ex.2 Find the side of a square whose area is 25 sq. m.
Sol. Now we find the number which when multiplied by itself gives us 25.
clearly, this number is 5.
∴ Side of the square = 5m.
Ex.3 In given figure, find the area of the shaded portion when all dimensions are given in centimeters.
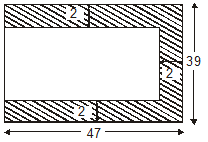
Sol. Length of the bigger rectangle = 47 cm
Breadth of the bigger rectangle = 39 cm
Area of the bigger rectangle = length × breadth
= (47 × 39) sq cm = 1833 sq cm
Length of the smaller rectangle = (47 –2) cm = 45 cm
Breadth of the smaller rectangle = (39 – 2– 2) cm = 35 cm
Area of the smaller rectangle = (45 × 35) sq cm
= 1575 sq cm
Area of shaded portion = (1833 – 1575) sq cm
= 258 sq cm
Ex.4 In given figure, if the area of the triangle ABC is 36cm2 and the height AD is 3 cm then the base would be
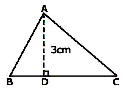
Sol. Given area = 36 sq cm and height = 3 cm base = ?
Area of the triangle = (base x height) ¸ 2
36 = (base × 3) ¸ 2
Base = (36 × 2) ¸ 3 = 24 cm
Ex.5 Bob wants to cover the floor of a room 3 m wide and 4 m long by squared tiles. If each square tile is of side 0.5 m, then find the number of tiles required to cover the floor of the room.
Sol.: Total area of tiles must be equal to the area of the floor of the room.
Length of the room = 4 m
Breadth of the room = 3 m
Area of the floor = length × breadth
= 4 m × 3 m = 12 sq m
Area of one square tile = side × side
= 0.5 m × 0.5 m
= 0.25 sq m
![]()
Ex.6 Find the area in square metre of a piece of cloth 1m 25 cm wide and 2 m long.
Sol. Length of the cloth = 2 m
Breadth of the cloth= 1 m 25 cm = 1 m + 0. 25 m = 1.25 m (since 25 cm = 0.25m)
Area of the cloth = length of the cloth × breadth of the cloth = 2 m × 1.25 m = 2.50 sq m
Solid Figures
- Books Name
- CBSE Class 6 Mathematics Book
- Publication
- Param Publication
- Course
- CBSE Class 6
- Subject
- Mathmatics
Solid Figures
A figure which have three dimensions as length, breadth and height is not a plane figure and we can not draw such figures on black board exactly. These three dimensional figures are called solids for example Cube, cuboid, cylinder cone, sphere etc. are some three dimensional figures. In this section we will learn how we determine the surface area of such solids.
1. Cuboid: A solid like an ordinary brick, each face of which is rectangle is called a rectangular solid or cuboid.
A cuboid has 6 rectangular plane surfaces, called faces. It has 12 edges and 8 vertices. If a cuboid is l cm long b cm broad and h cm high, then
(i) Total Surface Area of Cuboid: We know that the outer surface of cuboid is made up of six rectangles and the area of rectangle can be found by multiplying length by breadth.
Area of I rectangle = l × h
Area of opposite of I rectangle = l × h
Area of II rectangle = b × h
Area of opposite of II rectangle = b × h
Area of III rectangle = l × b
Area of opposite of III rectangle = l × b
Surface Area of a cuboid = Area of 6 rectangles = lb + lb + bh + bh + hl + hl = 2lb + 2bh + 2hl = 2(lb + bh + hl)
Total surface area of a cuboid = 2(lb + bh + hl)
(ii) Lateral surface area of cuboid = 2(l + b) × h cm2
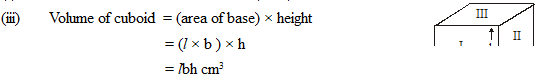
2. Cube: It is a particular case of a cuboid where length = breadth = height. Each edge of a cube is called its side.
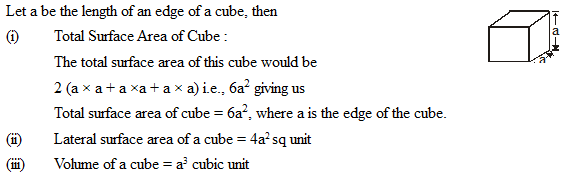
Ex.1 If length and breadth of a cuboid is 4 cm and 6 cm and having total surface area is 208 cm2 then find the height of cuboid.
Sol. Let L = 4 cm, B = 6 cm
Total surface area of cuboid = 2(LB + BH + HL)
208 = 2 [4 ´ 6 + 6 ´ H + H ´ 4]
208 = 2 [24 + 6 ´ H + H ´ 4]
208 = 2 [24 + 10H]
24 + 10 H = = 104
10 H = 104 – 24 = 80
H = = 8
there for height of the cuboid = 8 cm
Ex.2 A cube of 9 cm edge is immersed completely in a rectangular vessel containing water. If the dimensions of the base are 15 cm and 12 cm. Find the rise in water level in the vessel.
Sol. We have
Edge of the given cube = 9 cm.
∴ Volume of the cube = (9)3 cm3 = 729 cm3
If the cube is immersed in the vessel, then the water level rises. Let the rise in water level be h cm.
Clearly,
Volume of the cube = Volume of the water replaced by it.


 Carrier Point
Carrier Point
 ReginaTagebücher
ReginaTagebücher
