States of Matter
- Books Name
- Kaysons Academy Chemistry Book
- Publication
- Kaysons Publication
- Course
- JEE
- Subject
- Chemistry
STATES OF MATTER
There are five known phases, or states, of matter: solids, liquids, gases, and plasma and Bose-Einstein condensates.
Here we will study only three. Solid, Liquid and Gas

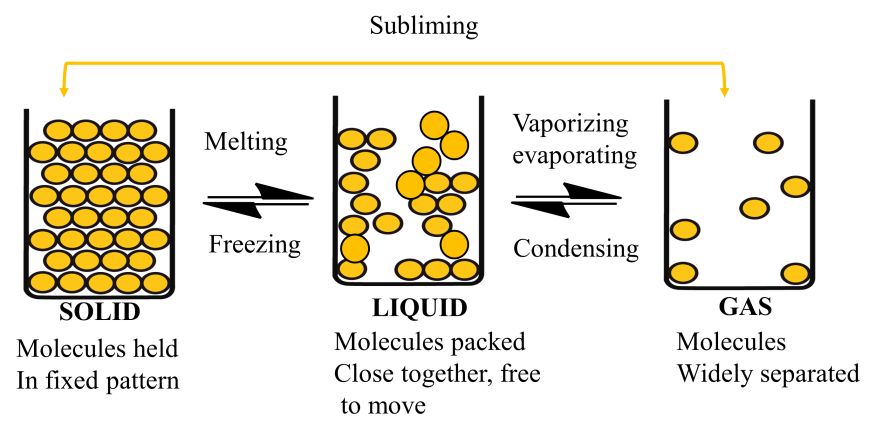

GASEOUS STATE
Only eleven elements exist as gases under normal conditions
GASEOUS STATE
The gaseous state is characterized by the following physical properties.
• Gases are highly compressible.
• Gases exert pressure equally in all directions.
• Gases have much lower density than the solids and liquids.
• The volume and the shape of gases are not fixed. These assume volume and shape of the container.
• Gases mix evenly and completely in all proportions without any mechanical aid (Diffusion)
Gas Laws
1. Boyle’s Law: At constant temperature
![]()
![]()
![]()
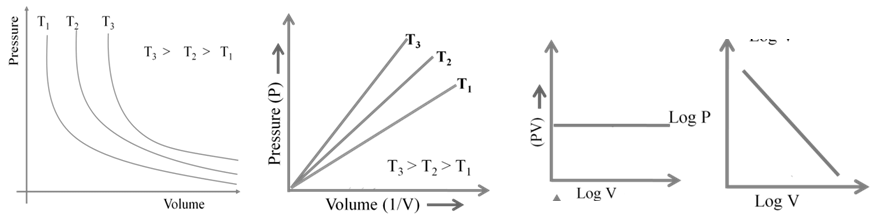
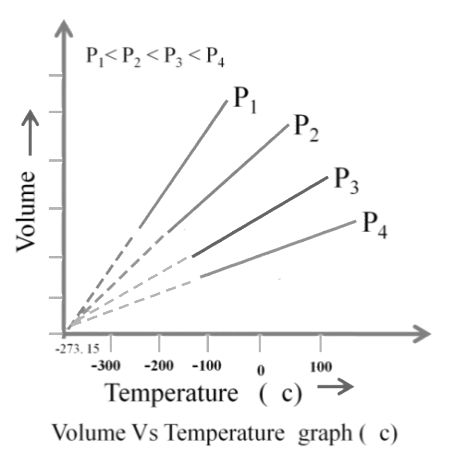
2. Charles Law: At constant pressure
![]()
Here K is a constant that depends on the pressure of gas, the amount of gas and also unit of volume if V1 and T1 are the initial values of volume and temperature of a gas then,
![]()
Also, if the temperature is now changed to T2 such that the volume change to V2
We can write,
![]()
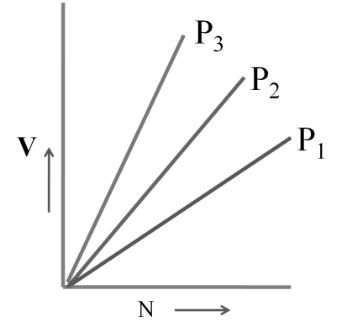
3. Avogadro's Law: At constant pressure and temperature
V µ n (no. of moles)
4. Ideal gas equation
Ideal gas equation
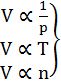
![]()
![]()
R = gas constant
w = mass of gas ρ = density
M = Mol weight of gas R = gas constant
![]()
![]()
5. Modified gas equation
![]()
![]()
![]()
![]()
Gas Constant ‘R’
![]()
= 0.0821 lit atm/ K. mole
= 8.314x 107 erg/k. mole
= 8.314 J/k. mole
= 1.987 cal/k. mole
Units and conversion
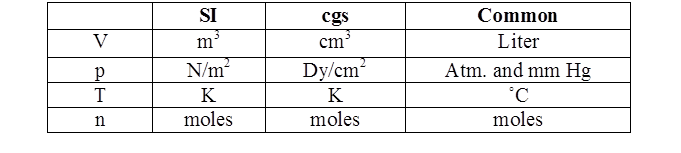
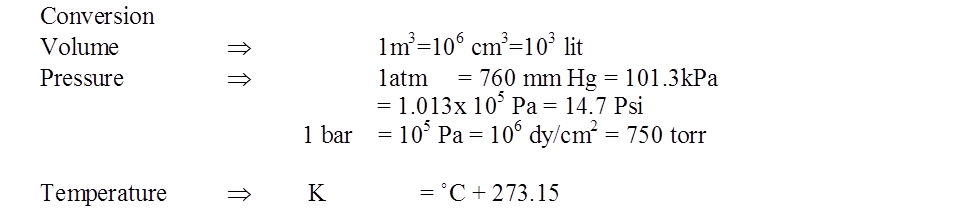
Dalton's law
- Books Name
- Kaysons Academy Chemistry Book
- Publication
- Kaysons Publication
- Course
- JEE
- Subject
- Chemistry
DALTON’S LAW

Dalton's law (also called Dalton's law of partial pressures) states that in a mixture of non-reacting ideal gases, the total pressure exerted is equal to the sum of the partial pressures of the individual gases.
KINETIC THEORY OF GASES
- Assumptions or postulates of the kinetic molecular theory of gases are given below.
- Gases consist of large number of identical particles (atoms or molecules) that are so small and so far apart on the average that the actual volume of the molecules is negligible in comparison to the empty space between them
- There is no force of attraction or repulsion between the particles of a gas
- Particles of a gas are always in constant and random motion.
- Collisions of gas molecules are perfectly elastic.
- At any particular time, different particles in the gas have different speeds and hence different kinetic energies
We can derive from kinetic theory they of gases
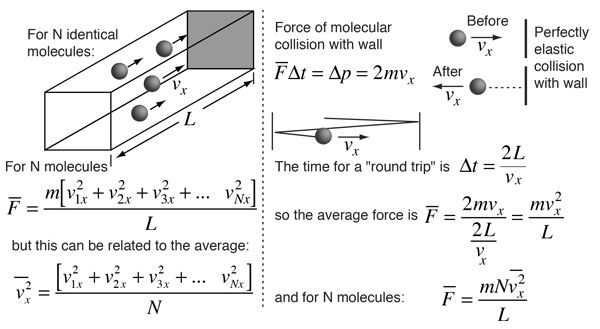
A gas molecule has velocity v and can be broken into thro components vx, vy and vz.
Travelling in x-axis momentum = mvx change in momentum after collision = - 2mvx. If gone back of comes back after time ‘t’
![]()
No. of collies is one second![]()
Change in momentum per second ![]()
Change in momentum on all three axis ![]()
![]()
![]()
![]()
For one mole of gas n = N and m x N = M (Mol. Wt.)
![]()
![]()
Kinetic Energy ![]() for one mole gas n = N and PV = RT
for one mole gas n = N and PV = RT
![]()
![]()
![]()
So kinetic energy, depends only on temperature
For one molecules ![]()
k = Boltzman constant
![]()
![]()
m = mass of gas particle
n = no. of gas particle
Vrms = root mean square velocity
PV = ![]() m. n. V2rms
m. n. V2rms
K.E. = ![]() RT for one mole of gas
RT for one mole of gas
K.E. = ![]() kT for one molecule where
kT for one molecule where
k = Boltzman constant =![]()
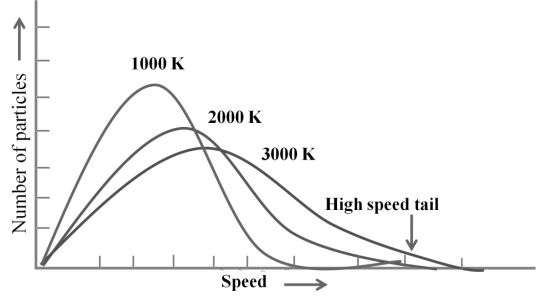
MAXWELL’S DISTRIBUTION OF SPEED AT DIFFERENT TEMPERATURE
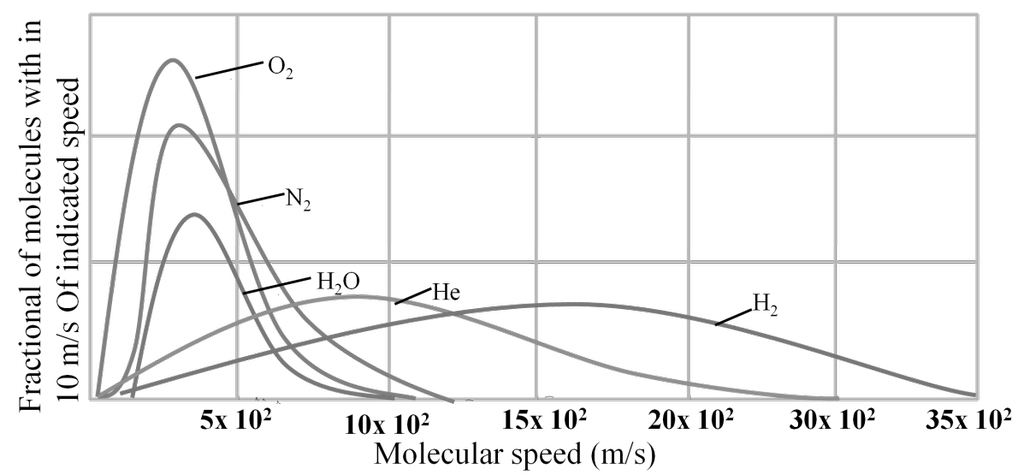
MAXWELL’S DISTRIBUTION OF SPEED FOR DIFFERENT GASES
DIFFERENT VELOCITIES FOR GASES
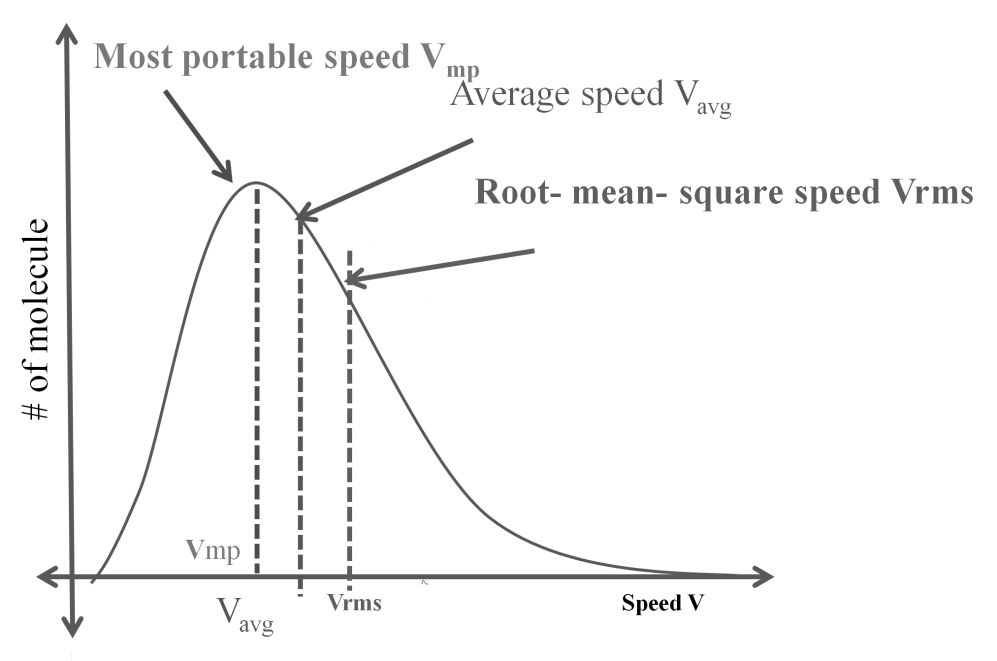
- The first typical velocity is the easiest to calculate and termed as the most probable velocity VMP. The velocity at the top of the curve is the most probable velocity as the largest number of molecules have this velocity.
- Average velocity of gas is simply the mean of all gas speeds possessed by all gas particles.

- Root mean square velocity is the square root of the mean of velocity squares VRMS =

DIFFERENT VELOCITIES FOR GASES
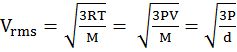
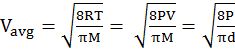
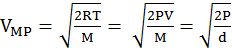
IMPORTANT SHORTCUT
Vrms: Vavg: VMP = 1.224 :1.128:1 and = 1: 0.92 :0.816
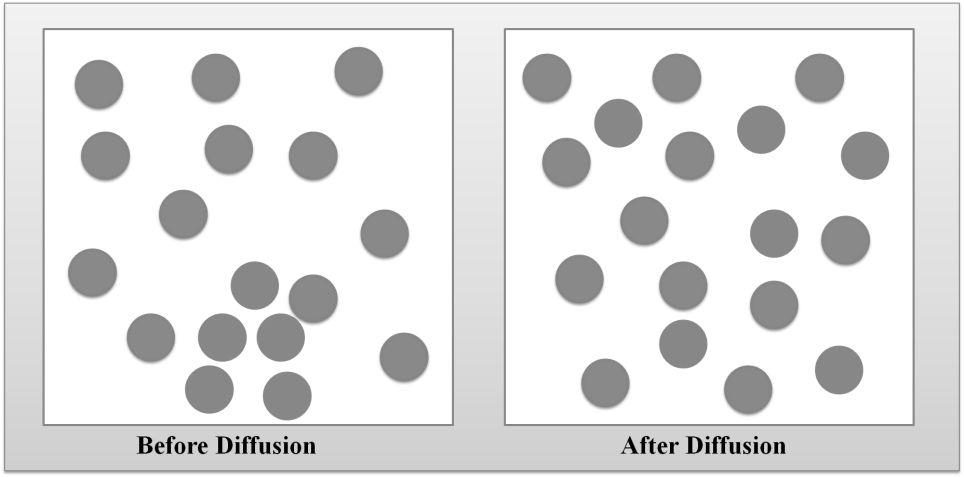
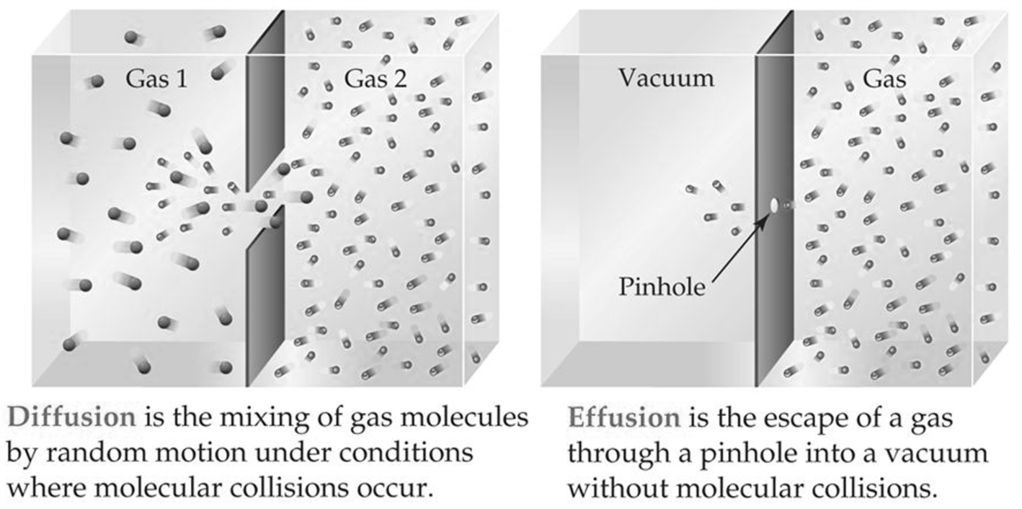
DIFFUSION OF GASES
DIFFUSION VS. EFFUSION
Diffusion- The tendency of the molecules of a given substance to move from regions of higher concentration to regions of lower concentration.
Examples: A sent spreading throughout a room or people entering a theme park.
Effusion: The process by which gas particles under pressure pass through a tiny hole.
Example: Air slowly leaking out of a tire or helium leaking out of a balloon.
Graham’s law of diffusion![]() when pressure is same.
when pressure is same.
Graham’s law of diffusionr![]() when pressure is also different
when pressure is also different
Real gas, Vanderwaal's Equation
- Books Name
- Kaysons Academy Chemistry Book
- Publication
- Kaysons Publication
- Course
- JEE
- Subject
- Chemistry
REAL GAS
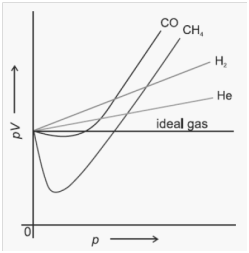
The Ideal gas equation shows big deviation at high pressures and low temperatures from ideal gas equation.
If you compress an Ideal gas to very high pressure when will it become a liquid? ????
Vanderwaal’s equation for real gas.
1. Volume correction. Actual volume available for gas to freely move is
VR = Vj – n b
b = Volume correction constant taking into size and gas molecule.
2. Pressure correction:
PReal = PIdeal – attractive forces between molecules.

PReal = Pideal – ![]()
A = Pressure correction
![]()
![]()
![]() . a, b are Vander Waals constant; n is the number of moles of gas
. a, b are Vander Waals constant; n is the number of moles of gas
V.W. Constants
‘b’ ![]() size of molecule
size of molecule
Thumb rule b = 4 x Volume and one atom/molecule.
‘a’ ![]() Inter molecule forces between atom/molecule gas
Inter molecule forces between atom/molecule gas
Ex: put in increasing order ‘a’ and ‘b’ for H2, He, NH3, O2
b = H2 < He < NH3 < O2
a = He < H2 < O2 < NH3
Compressibility factor Z
![]()
Z = 1 Þ Ideal gas. No. attraction/Repulsion
Z < 1 ÞReal gas. Attractive forces dominate
Z > 1 ÞReal gas. Repulsive forces dominate
UNIT OF ‘a’ AND ‘b’
![]()
![]()
Real gas behaves like ideal gas at low pressure and high temperature.
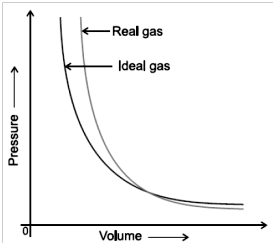
Boyle’s temp =![]() temperature at which Real gas behave like ideal gas.
temperature at which Real gas behave like ideal gas.
Liquefaction of Gas
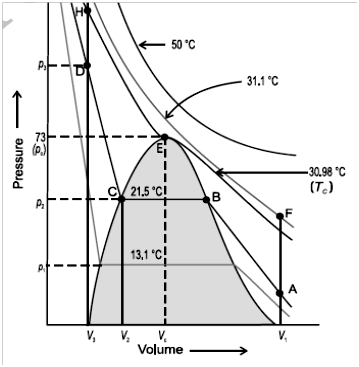
Critical temperature (TC) is the temperature above which a gas cannot be liquefied however high pressure may be.
Critical pressure (PC) is minimum pressure required to liquefy gas at the critical temperature.
Critical volume (VC) is the volume occupied by one mole of gas at critical temperature and critical pressure.
![]()
For gas CO2
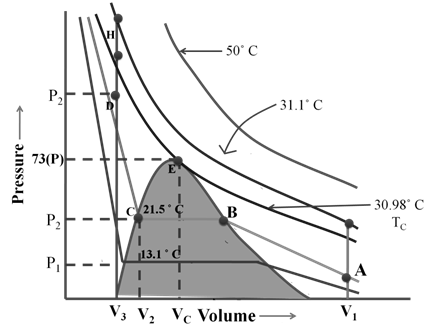
Real gases can only be liquefied by increasing pressure, if the temperature is below critical temperature
Here Vc and Pc volume and pressure at critical temperature when it touches the curve.
Surface tension, Viscosity
- Books Name
- Kaysons Academy Chemistry Book
- Publication
- Kaysons Publication
- Course
- JEE
- Subject
- Chemistry
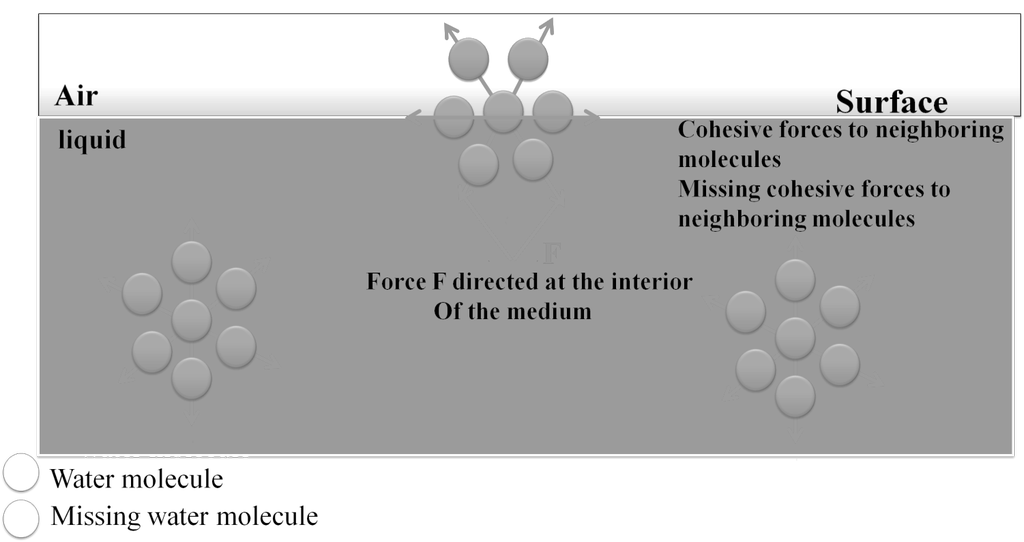
SURFACE TENSION
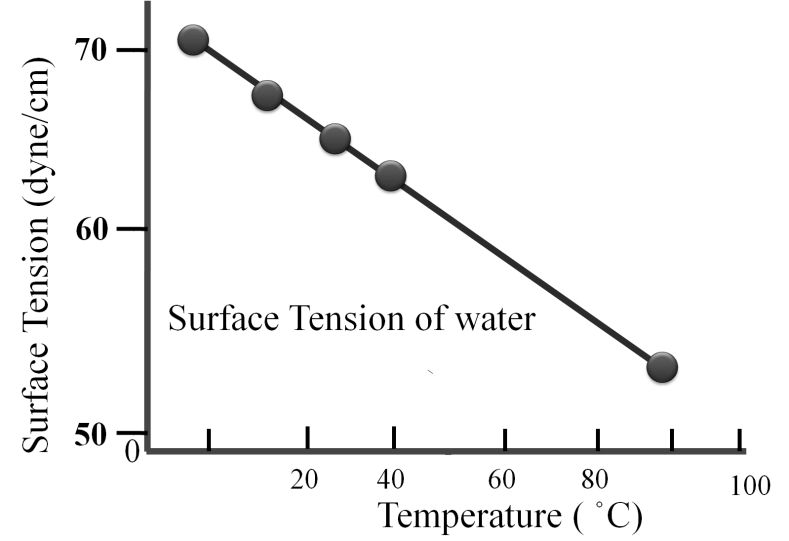
Surface tension is defined as the force acting per unit length perpendicular to the line drawn on the surface of liquid. It is denoted by Greek letter γ (Gamma). It has dimensions of kg s-2 and in SI unit it is expressed as N m-1. Surface tension reduces with increase in temperature.
SURFACE TENSION VS. TEMPERATURE PLOT
VISCOSITY
It is one of the characteristic properties of liquids. Viscosity is a measure of resistance to flow which arises due to the internal friction between layers of fluid as they slip past one another while liquid flows.

F ![]() A (A is the area of contact)
A (A is the area of contact)
F ![]() (where,
(where,![]() is velocity gradient)
is velocity gradient)
![]()
‘n’ is proportionality constant and is called coefficient of viscosity.
Viscosity coefficient is the force when velocity gradient is unity and the area of contact is unit area.
SI unit of viscosity coefficient is Newton second per square meter (N s m-2) = Pascal second (Pa s = 1kg m-1s-1). In cgs system the unit of coefficient of viscosity is poise
1 poise = 1 g cm-1s-1 = 10-1kg m-1s-1
IMPORTANT FORMULA
- Ideal gas PV = nRT =
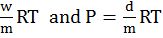
- Graham’s law of diffusion
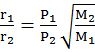
- Separation by diffusion f=n1'n2'n1n2=AfterBefore f1=M2M1=one stage separation f1=f here x=no. of shape
 Kinetic Theory PV = 1/3 mnv2rms
Kinetic Theory PV = 1/3 mnv2rms - Kinetic Energy/mole = 3/2 RT
- Kinetic Energy/molecule = 3/2 kT k(Boltzmann constant) =R/NA
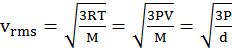

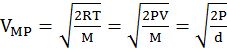
- vrms: vavg: vMP = 1.224 :1.128:1 = 1: 0.92 :0.816
- Vanderwaal’s Gas
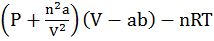
- Boyles temp Tb =

- Critical constants
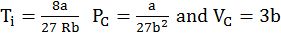
- Inversion temperature

- Cp – CV = R and CP/CV = g
- Compression factor Z =
 Z = 1 for ideal gas (Z < 1 lower P Z > 1 higher P)
Z = 1 for ideal gas (Z < 1 lower P Z > 1 higher P) - Cp – Cv = R and Cp/Cv = g
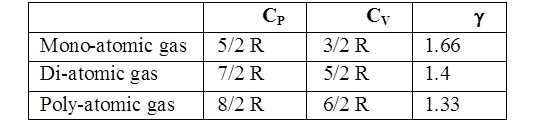

 Kaysons Publication
Kaysons Publication
