- Books Name
- AMARENDRA PATTANAYAK Mathmatics Book
- Publication
- KRISHNA PUBLICATIONS
- Course
- CBSE Class 11
- Subject
- Mathmatics
Trigonometric Functions of Sum and Difference of Two Angles:
Consider the following figure:
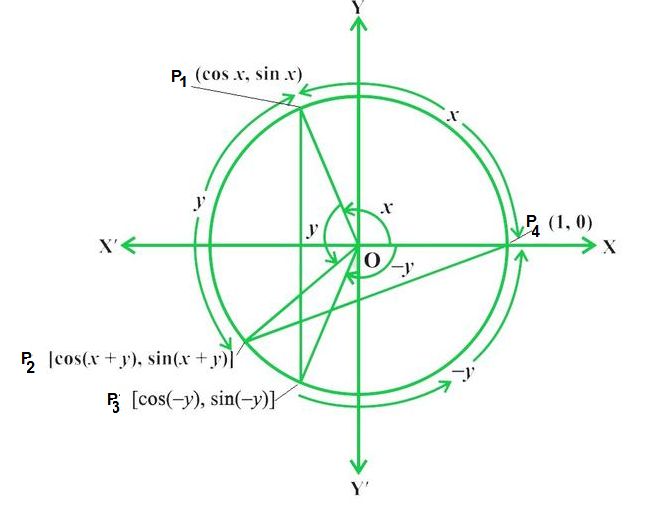
A circle is drawn with center as origin and radius 1 unit. A point P1 is chosen at an angle of x units from x-axis. The co-ordinates are mentioned in the figure. Another point P2 is chosen, at an angle of y units from the line segment OP1. P3 is a point on the circle which is at an angle of y units from x-axis, measured clockwise.
Now, in the given figure, Δ OP1P3 is congruent to Δ OP2P4, by SAS congruency criteria.
Hence, P1P3 = P2P4 (CPCT)
⇒(P1P3 )2= (P2P4)2
Since we know the coordinates of all the four points, hence using distance formula, we can write:
[cos x – cos (-y)]2 + [sin x – sin (-y)]2 = [1- cos (x+y)]2 + sin2 (x+y)
On solving the above equation, we have the following identity:
Sum Formula for Cosine cos(x + y) = cos x cos y – sin x sin y ……… (1)
Replacing y by -y in identity (1), we get,
cos(x – y) = cos x cos y + sin x sin y …….… (2)
Also,
cos (π/2 – x) = sin x ……………… (3)
That can be obtained by replacing x by π/2 and y by x in identity (2). Also,
sin (π/2 – x) = cos x………….…… (4)
As, sin (π/2 – x) = cos [π/2 – (π/2 – x)] (using identity 3). So,
sin (π/2 – x) = cos x
Now we have the idea about the expansion of sum and difference of angles of cos. Now let us try to use it for finding the values of sum and difference of angles of sin.
sin (x + y) can be written as cos [π/2 – (x + y)] which is equal to cos [(π/2 – x) – y]
Now, using identity (2) we can write,
cos [(π/2 – x) – y] = cos (π/2 – x) cos y + sin (π/2 – x) sin y
= sin x cos y + cos x sin y
Hence,
Sum Formula for Sine sin (x + y) = sin x cos y + cos x sin y …………………………. (5)
Replace y by –y in the above formula, we get
sin (x – y) = sin x cos y – cos x sin y .…………………………….. (6)
Now if we substitute suitable values in identities (1), (2), (5) and (6), we have the following:
cos (π/2 + x) = -sin x
sin (π/2 + x) = cos x
cos (π± x) = – cos x
sin (π – x) = sin x
sin (π + x) = – sin x
sin (2π – x) = -sin x
cos (2π – x) = cos x
After having a brief idea about the expansion of sum and difference of angles of sin and cos, the expansion for tan and cot is given by
tan (x + y) = (tan x + tan y)/ (1-tan x tan y)
tan (x – y) = (tan x – tan y)/ (1+tan x tan y)
Similarly;
cot (x + y) = (cot x cot y – 1)/(cot y + cot x)
cot (x – y) = (cot x cot y + 1)/(cot y – cot x)
EXTRA:
Generalisation :
- sin (A1 + A2 + ...... + An) = cos A1 cos A2 ...... cos An (S1 – S3 + S5 – ....)
- cos (A1 + A2 + ...... + An) = cos A1 cos A2 ...... cos An (1 – S2 + S4 – S6 + ....)
- tan (A1 + A2 + ...... + An) =
where S1 = Σ tan A , S2 = Σ tan A1 tan A2,
S3 = Σ tan A1 tan A2 tan A3 and so on.
FORMULAS TO TRANSFORM PRODUCTS INTO SUM AND DIFFERENCE
- 2 sin A cos B = sin(A+B) + sin (A – B)
- 2 cos A sin B = sin (A+B) – sin (A – B)
- 2 cos A cos B = cos (A+B) + cos (A – B)
- 2 sin A sin B = cos (A – B) – cos (A + B)
FORMULAS TO TRANSFORM SUM OR DIFFERENCE INTO PRODUCT
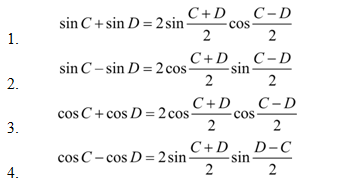
TRIGONOMETRIC FUNCTIONS OF MULTIPLE AND SUB-MULTIPLE ANGLES
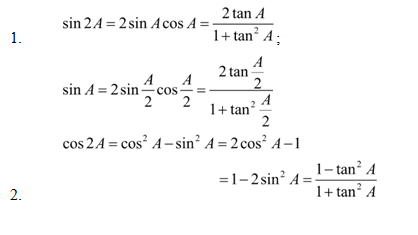
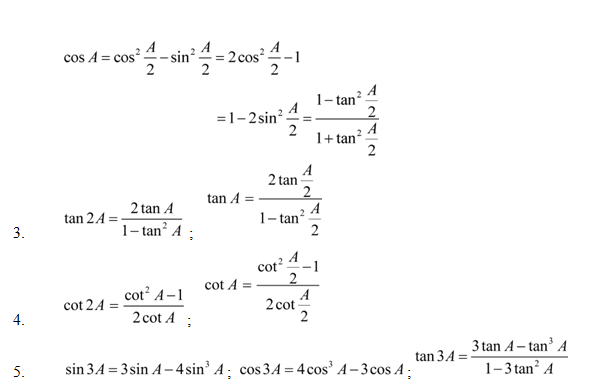
FORMULAS FOR LOWERING THE DEGREE OF TRIGONOMETRIC FUNCTIONS

CONDITIONAL TRIGONOMETRIC IDENTITIES
If A + B + C = 180° (or π), or A, B, C are angles of a triangle. Then
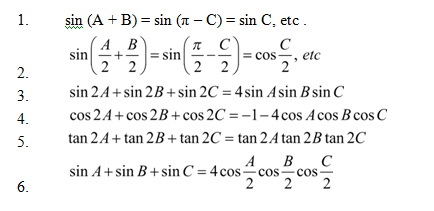
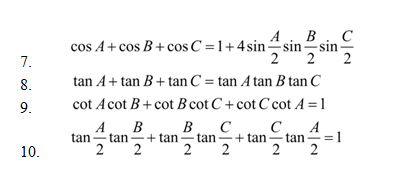

 KRISHNA PUBLICATIONS
KRISHNA PUBLICATIONS
