Introduction

Chapter -4

TRIANGLES

Introduction

In Class IX, you have seen that all circles with the same radii are congruent, all squares with the same side lengths are congruent and all equilateral triangles with the same side lengths are congruent.
Now consider any two (or more) circles [see Fig (i)]. Are they congruent? Since all of them do not have the same radius, they are not congruent to each other. Note that some are congruent and some are not, but all of them have the same shape. So they all are, what we call, similar. Two similar figures have the same shape but not necessarily the same size. Therefore, all circles are similar. What about two (or more) squares or two (or more) equilateral triangles [see Fig (ii) and (iii)]? As observed in the case of circles, here also all squares are similar and all equilateral triangles are similar.

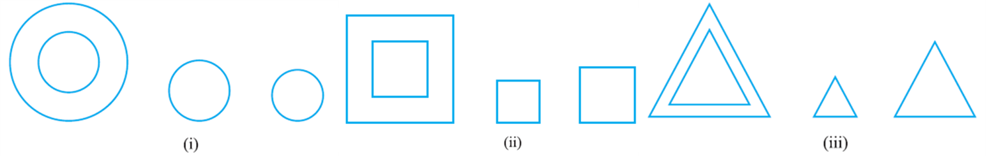
From the above, we can say that all congruent figures are similar but the similar figures need not be congruent. Can a circle and a square be similar? Can a triangle and a square be similar? These questions can be answered by just looking at the figures (see Fig). Evidently these figures are not similar. (Why?)
What can you say about the two quadrilaterals ABCD and PQRS (see Fig.1)? Are they similar? These figures appear to be similar but we cannot be certain about it. Therefore, we must have some definition of similarity of figures and based on this definition some rules to decide whether the two given figures are similar or not. For this, let us look at the photographs given in Fig. 2:

You will at once say that they are the photographs of the same monument (Taj Mahal) but are in different sizes. Would you say that the three photographs are similar? Yes, they are.
What can you say about the two photographs of the same size of the same person one at the age of 10 years and the other at the age of 40 years? Are these photographs similar? These photographs are of the same size but certainly they are not of the same shape. So, they are not similar.
What does the photographer do when she prints photographs of different sizes from the same negative? You must have heard about the stamp size, passport size and postcard size photographs. She generally takes a photograph on a small size film, say of 35mm size and then enlarges it into a bigger size, say 45mm (or 55mm). Thus, if we consider any line segment in the smaller photograph (figure), its corresponding line segment in the bigger photograph (figure) will be    of that of the line segment. This really means that every line segment of the smaller photograph is enlarged (increased) in the ratio 35:45 (or 35:55). It can also be said that every line segment of the bigger photograph is reduced (decreased) in the ratio 45:35 (or 55:35). Further, if you consider inclinations (or angles) between any pair of corresponding line segments in the two photographs of different sizes, you shall see that these inclinations (or angles) are always equal. This is the essence of the similarity of two figures and in particular of two polygons. We say that:

Similarity of Triangles
You may recall that triangle is also a polygon. So, we can state the same conditions for the similarity of two triangles. That is:
Two triangles are similar, if
(i) Their corresponding angles are equal and
(ii) Their corresponding sides are in the same ratio (or proportion).
Note that if corresponding angles of two triangles are equal, then they are known as equiangular triangles. A famous Greek mathematician Thales gave an important truth relating to two equiangular triangles which is as follows: The ratio of any two corresponding sides in two equiangular triangles is always the same. It is believed that he had used a result called the Basic Proportionality Theorem (now known as the Thales Theorem) for the same.

Theorem: If a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio.
Proof: We are given a triangle ABC in which a line parallel to side BC intersects other two sides AB and AC at D and E respectively (see Fig).

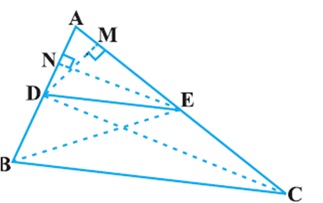
We need to prove that   

Let us join BE and CD and then draw DM ⊥ AC and EN ⊥ AB.
  
Recall from Class IX, that area of ΔADE is denoted as are

  
  
  
  
  
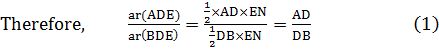
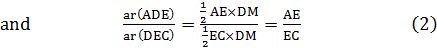
Note that ∆BDE and DEC are on the same base DE and between the same parallels BC and DE.
So,                     ar(BDE) = ar(DEC)
Therefore, from (1), (2) and (3), we have: