- Books Name
- Science Made Easy Science Book
- Publication
- Science Made Easy
- Course
- CBSE Class 9
- Subject
- Science
Gravitational Acceleration and its value at the surface of earth
→ The uniform acceleration produced in a freely falling object due to the gravitational force of
earth, is called acceleration due to gravity.
→ It is represented by ‘g’ and it always acts towards the centre of the earth.
Mathematical Expression for g
From the second law of motion, force is the product of mass and acceleration.
F = ma
For free fall, acceleration is replaced by acceleration due to gravity.
Therefore, force becomes:
F = mg ….(i)
But from Universal Law of Gravitation,
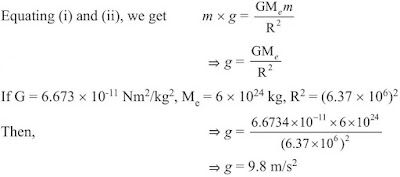
Factors Affecting the Value of g
- As the radius of the earth increases from the poles to the equator, the value of g becomes greater at the poles than at the equator.
- As we go at large heights, value of g decreases.
To Calculate the Value of g
Value of universal gravitational constant, G = 6.7 × 10–11 N m2/ kg2,
Mass of the earth, M = 6 × 1024 kg, and
Radius of the earth, R = 6.4 × 106 m
Putting all these values in equation (iii), we get:
![]()
Thus, the value of acceleration due to gravity of the earth, g = 9.8 m/s2.
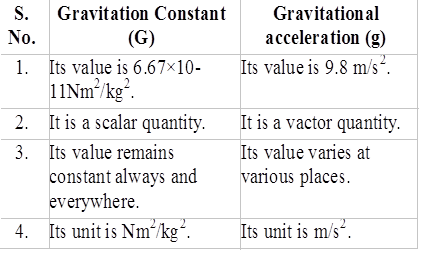
Difference between Gravitation Constant (G) and Gravitational Acceleration (g)
Motion of Objects Under the Influence of Gravitational Force of the Earth
Let an object is falling towards earth with initial velocity u. Let its velocity, under the effect of gravitational acceleration g, changes to v after covering the height h in time t.
Then the three equations of motion can be represented as:
Velocity (v) after t seconds, v = u + ght
Height covered in t seconds, h = ut + ½gt2
Relation between v and u excluding t, v2 = u2 + 2gh
The value of g is taken as positive in case of the object is moving towards earth and taken as negative in case of the object is thrown in opposite direction of the earth.

 Science Made Easy
Science Made Easy
