- Books Name
- CBSE Class 6 Mathematics Book
- Publication
- Param Publication
- Course
- CBSE Class 6
- Subject
- Mathmatics
Circle
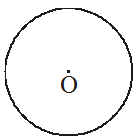
A circle is a simple closed curve all of whose points are at the same distance from a given point O in the same plane . The given point O is called the centre of the circle.
It has some very special properties.
Parts of a circle
Here is a circle with centre C (Fig ) A, P, B, M are points on the circle. We will see that
CA = CP = CB = CM.
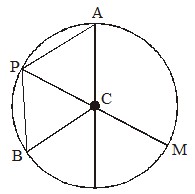
Each of the segments CA , CP , CB, CM is radius of the circle. The radius is a line segment that connects the centre to a point on the circle. CP and CM are radii (plural of ‘radius’) such that C, P, M are in a line. PM is known as diameter of the circle. Diameter is a double the size of a radius.
PB is a chord connecting two points on a circle.
An arc is a portion of circle.
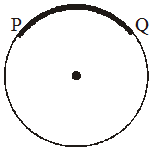
If P and Q are two points we get the arc PQ. We write as . ![]()
As in the case of any simple closed curve we can think of the interior and exterior of a circle. A region in the interior of a circle enclosed by an arc on one side and a pair of radii on the other two sides is called a sector.
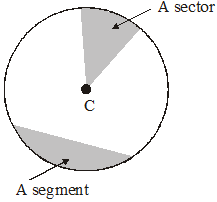
A region in the interior of a circle enclosed by a chord and an arc is called a segment of the circle. The distance around a circle is called its circumference.

 Param Publication
Param Publication
