Angular momentum

ANGULAR MOMENTUM

(ii)  Angular Momentum of a Rigid Body Rotation about a Fixed Axis

Uniform Pure Rolling

 v_p = v_Q

 v - Rw = 0

 v = Rw

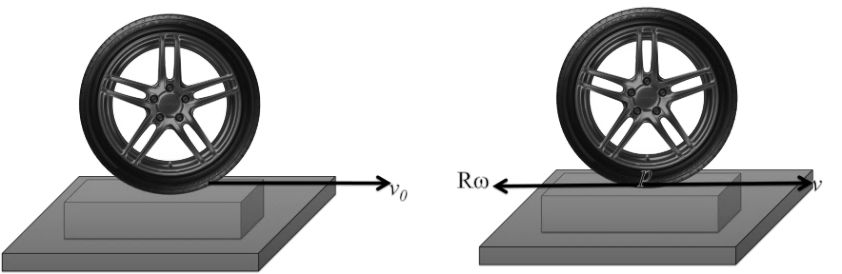
If vp > v_Q or v > Rw, the motion is said to be forward slipping and if vp < v_Q or v < Rw, the motion is said the backward slipping

Accelerated  Pure Rolling

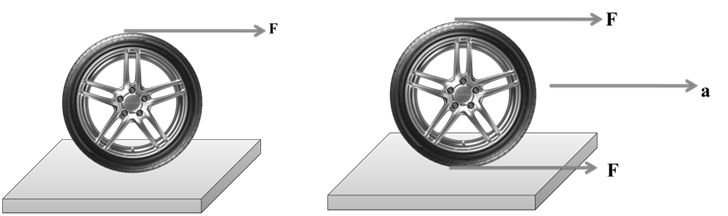
v = Rw

a_t = Ra

 

 

 

 

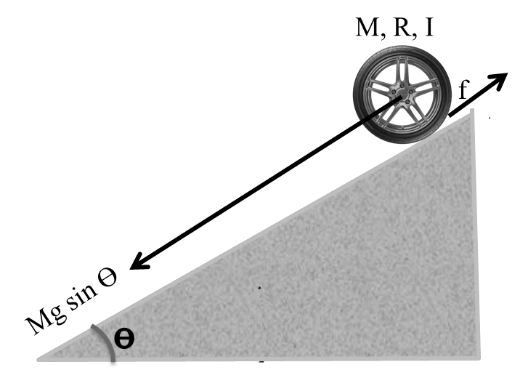
Rolling on Rough Incline plane

 

  

  

   

     

I_solid  <  I_hollow  or  a_solid  > a_hollow

t_solid   <   t_hollow

   

Case

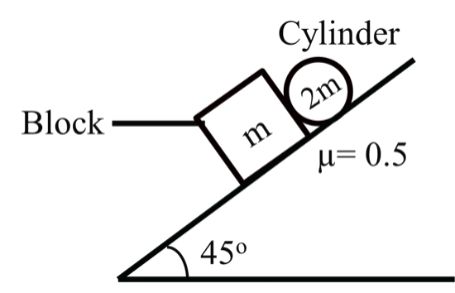
A block of mass m and a cylinder of mass 2m are released on a rough inclined plane, inclined at an angle  with the horizontal. The coefficient of friction for all the contact surface is 0.5 find the accelerations of the block and the cylinder.

Assume pure rolling.

If the block and cylinder move independently on the incline, their acceleration are

 

 

 

 

 So, both the bodies will move in contact

with each other with common acceleration a which we have to calculate.

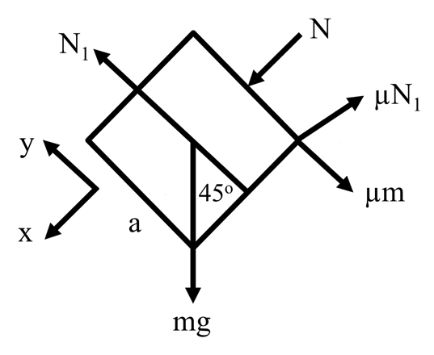
Free body diagram of block is as shown.

 

 

                          …..(i)

 

                                   ….(ii)

From Eqs. (i) and (ii), we get

 

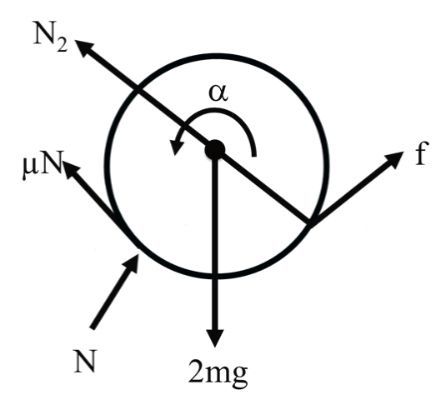
The free body diagram of cylinder is as shown.

 

 

And  

 

From Eqs. (iv) and (v), we get

 

Using this in Eq. (iii), we get

Case:- Ladder → mass = m, Length = L

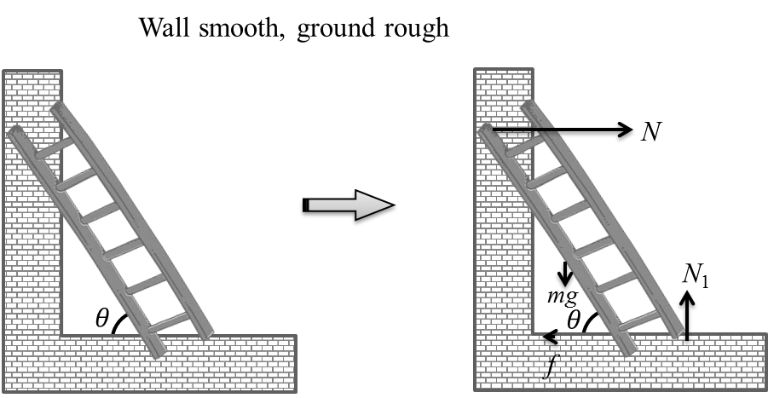
For equilibrium

f – N = 0

N₁ – mg = 0

Torque about C. M. of Ladder

    

Substitute value of N₁ and f

 

 

Case:- Topping and Sliding

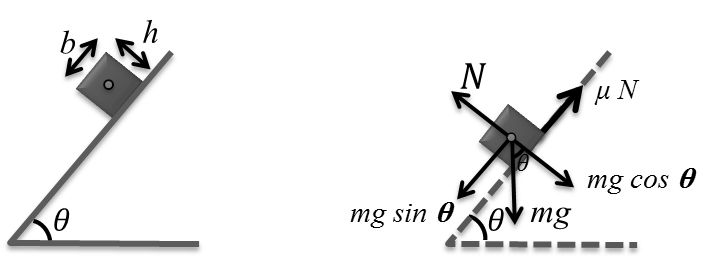
For translational equilibrium

  

 

For rotational equilibrium

  

  

 

 block has a tendency to slide before topple  

 block has a tendency to topple before slide 

 block slides down 

 block topples