5. Methods of Integration(Integration by Partial Fractions)

Integration by Partial  Fractions

If f(x) and g(x) are polynomial functions such function. that g(x) ≠ 0 then f(x)/g(x) is called Rational Functions. If degree f(x) < degree g(x) then f(x)/g(x) is called a proper rational function then apply partial fraction method. If degree f(x) < degree g(x) then f(x)/g(x) is called an improper rational function. If f(x)/g(x) is an improper rational function then by dividing f(x) by g(x), we can express f(x)/g(x) as the sum of a polynomial and a proper rational function, then apply partial fraction method.

Partial Fractions

Any proper rational function p(x)/q(x) can be expressed as the sum of rational functions, each having the simplest factor q(x), each such fraction is known as a partial function and the process of obtaining them is called the decomposition or resolving of the given function into partial fractions.

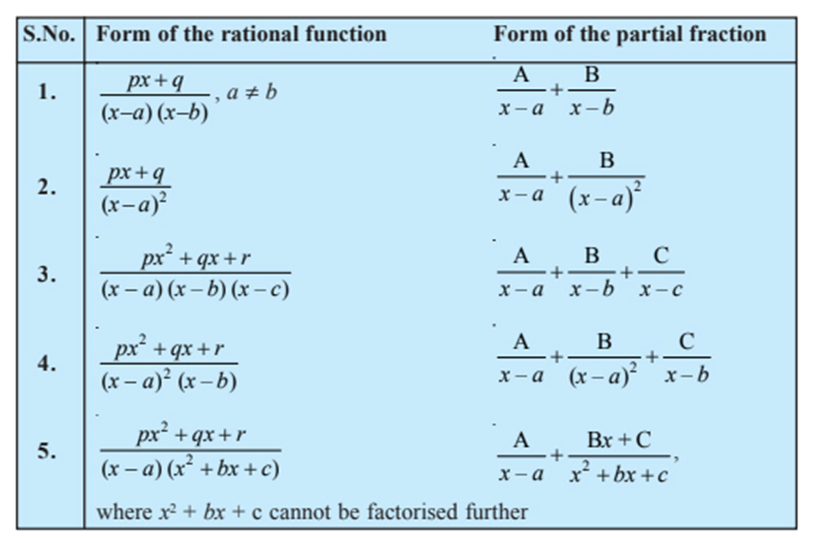
Example : Evaluate ʃ (x – 1)/(x + 1)(x – 2) dx

Solution:

Let (x – 1)/(x + 1)(x – 2) = A/(x + 1) + B/(x – 2)

Then, (x – 1) = A(x – 2) + B(x + 1) ………………(i)

Putting x = -1 in (i), we get A = 2/3

Putting x = 2 in (i), we get B = 1/3

Therefore,  

(x – 1)/(x + 1)(x – 2) = 2/3(x + 1) + 1/3(x – 2)

=> ʃ (x – 1)/(x + 1)(x – 2) = 2/3ʃdx/(x + 1) + 1/3ʃdx/(x – 2)

                                       = 2/3log | x + 1 | + 1/3 log | x – 2 | + C

Example : Evaluate ʃdx/(x³ + x² + x + 1)

Solution:

We have 1/(x³ + x² + x + 1) = 1/x²(x + 1) + (x + 1) = 1/(x + 1)(x² + 1)

Let 1/(x + 1)(x² + 1) = A/(x + 1) + Bx + C/(x² + 1)  ……………………(i)

=> 1 ≡ A(x² + 1) + (Bx + C) (x + 1)

Putting x = -1 on both sides of (i), we get A = 1/2.

Comparing coefficients of x² on the both sides of (i), we get

A + B = 0 => B = -A = -1/2

Comparing coefficients of x on the both sides of (i), we get

B + C = 0 => C = -B = 1/2

Therefore, 1/(x + 1) (x² + 1) = 1/2(x + 1) + (-1/2x + 1/2)/(x² + 1)

Therefore, ʃ1/(x + 1) (x² + 1) = ʃdx/(x + 1) (x² + 1)

                                              = 1/2ʃdx/(x + 1) – 1/2ʃx/(x² + 1)dx + 1/2ʃdx/(x² + 1)

                                              = 1/2ʃdx/(x + 1) – 1/4ʃ2x/(x² + 1)dx + 1/2ʃdx/(x² + 1)

                                              = 1/2 log | x + 1 | – 1/4 log | x² + 1 | + 1/2 tan^-1x + C

Example : Evaluate ʃx²/(x² + 2)(x² + 3)dx

Solution: 

Let x²/(x² + 2) (x² + 3) = y/(y + 2)(y + 3) where x² = y.

Let y/(y + 2) (y + 3) = A/(y + 2) + B/(y + 3)

=> y ≡ A(y + 3) + B/(y + 2)   ………………(i)

Putting y = -2 on the both sides of (i), we get A = -2.

Putting y = -3 on the both sides of (i), we get B = 3.

Therefore, y/(y + 2) (y + 3) = -2/(y + 2) + 3/(y + 3)

=> x²/(x² + 2) (x² + 3) = -2/(x² + 2) + 3/(x² + 3)

=> ʃx²/(x² + 2) (x² + 3) = -2ʃdx/(x² + 2) + 3ʃdx/(x² + 3)

                                     = -2/√2tan^-1(x/√2) + 3/√3tan^-1(x/√3) + C

                                     = -√2tan^-1(x/√2) + √3tan^-1(x/√3) + C

Example : Evaluate ʃdx/x(x⁴ + 1)?

Solution:

We have

I = ʃdx/x(x⁴ + 1) = ʃx³/x⁴ (x⁴ + 1) dx [multiplying numerator and denominator by x³].

Putting x⁴ = t and 4x³dx = dt, we get

I = 1/4ʃdt/t(t + 1)

  = 1/4∫{1/t – 1/(t + 1)}dt   [by partial fraction]

  = 1/4ʃ1/t dt – 1/4ʃ1/(t + 1)dt

  = 1/4 log | t | – 1/4 log | t + 1 | + C

  = 1/4 log | x⁴ | – 1/4 log | x⁴ + 1 | + C

  = (1/4 * 4) log | x | – 1/4 log | x⁴ + 1 | + C

  = log | x | – 1/4 log | x⁴ + 1 | + C