1. Imaginary Numbers and Powers
- Books Name
- AMARENDRA PATTANAYAK Mathmatics Book
- Publication
- KRISHNA PUBLICATIONS
- Course
- CBSE Class 11
- Subject
- Mathmatics
Chapter 5
Complex Numbers and Quadratic Equations
Imaginary Numbers and Powers
It is a solution to the quadratic equation or expression, x2+1 = 0, such as;
x2 = 0 – 1
x2 = -1
x = √-1
x = i
Therefore, an imaginary number is the part of complex number which we can write like a real number multiplied by the imaginary unit i, where i2 = -1. The imaginary number, when multiplied by itself, gives a negative value.
Value of Powers of i
We know, i2 = -1, let us calculate the value of ‘i’ raised to the power other imaginary numbers.
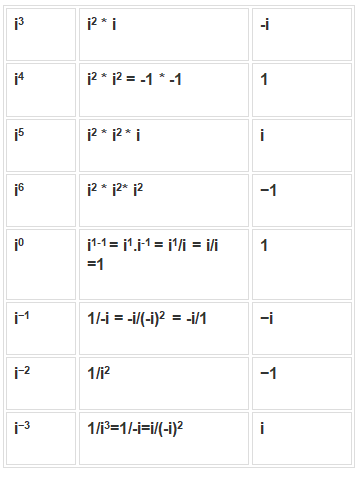
i4n = 1
i4n+1 = i
i4n+2= -1
i4n+3=-i
2. Complex Numbers and Algebra of Complex Numbers
- Books Name
- AMARENDRA PATTANAYAK Mathmatics Book
- Publication
- KRISHNA PUBLICATIONS
- Course
- CBSE Class 11
- Subject
- Mathmatics
Complex Numbers and Algebra of Complex Numbers
Example 1: Solve the complex number √-4?
Solution: √-4 = √(-1 * 4) = 2√-1 = 2i
Example 2: Solve y2+1 = 0
Solution: y2+1 = 0
y2 = -1
y = √-1 = i
Check:
L.H.S = y2+1 = i2 + 1 = -1 + 1 = 0 (R.H.S)
Example 3: Find the value of √-3 + 1.
Solution: √-3 + 1
= √-1 √3 + 1
i√3 + 1
Example 4: Solve x2+16 = 0
Solution: x2+16 = 0
x2= -16
x =√-16
x = i √16
x = i4
Check:
L.H.S = x2+16 = (i4)2 + 16 = i242 + 16 = -16 +16 = 0 (R.H.S)
Example 5 : Simplify.
(2i)(−6i)(−7i)(2i)(−6i)(−7i)
Rewrite the expression grouping the real and imaginary parts.
(2i)(−6i)(−7i)=(2)⋅(−6)⋅(−7)⋅i⋅i⋅i
=84⋅i⋅i⋅i(2i)(−6i)(−7i)
=(2)⋅(−6)⋅(−7)⋅i⋅i⋅i=84⋅i⋅i⋅i
Simplify the imaginary part using the property of multiplying powers.
=84i3=84i2i1=84i3=84i2i1
Recall the definition of i.
Since i2=−1:
=84(−1)i=−84i=84(−1)i=−84i
Example 6:Simplify the principal square root of √−64
Ans:
√−64
Taking the square root and substituting √-1= i
=(√−1). √64=√(−1)⋅ √64=i√64
=8i
3. The Modulus and the Conjugate of a Complex Numbers
- Books Name
- AMARENDRA PATTANAYAK Mathmatics Book
- Publication
- KRISHNA PUBLICATIONS
- Course
- CBSE Class 11
- Subject
- Mathmatics
The Modulus and the Conjugate of a Complex Numbers
Modulus of a complex number gives the distance of the complex number from the origin in the argand plane, whereas the conjugate of a complex number gives the reflection of the complex number about the real axis in the argand plane.
The product of two complex conjugate numbers is real. = a - ib. , which is real. Thus, the modulus of any complex number is equal to the positive square root of the product of the complex number and its conjugate complex number.
Conjugate of a Complex Number
Conjugate of a complex number z = x + iy is denoted by
![]() = x – iy. It is the reflection of the complex number about the real axis on Argand’s plane or the image of the complex number about the real axis on Argand’s plane. If we replace the ‘i’ with ‘- i’, we get conjugate of the complex number.
= x – iy. It is the reflection of the complex number about the real axis on Argand’s plane or the image of the complex number about the real axis on Argand’s plane. If we replace the ‘i’ with ‘- i’, we get conjugate of the complex number.
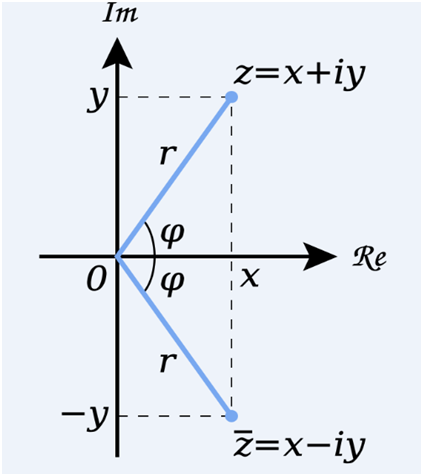
Geometrical representation of the complex number is shown in the figure given below:
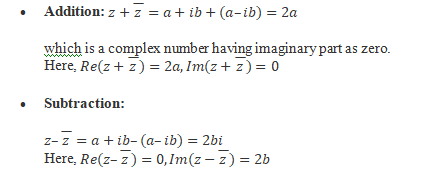
Properties of conjugate of a complex number:
If z, z1 and z2 are complex number, then
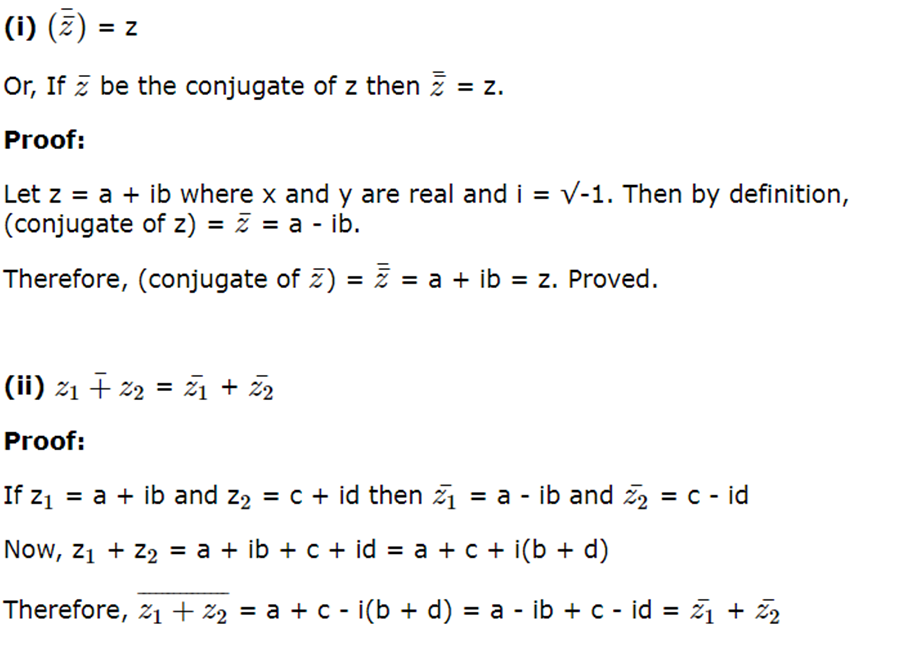
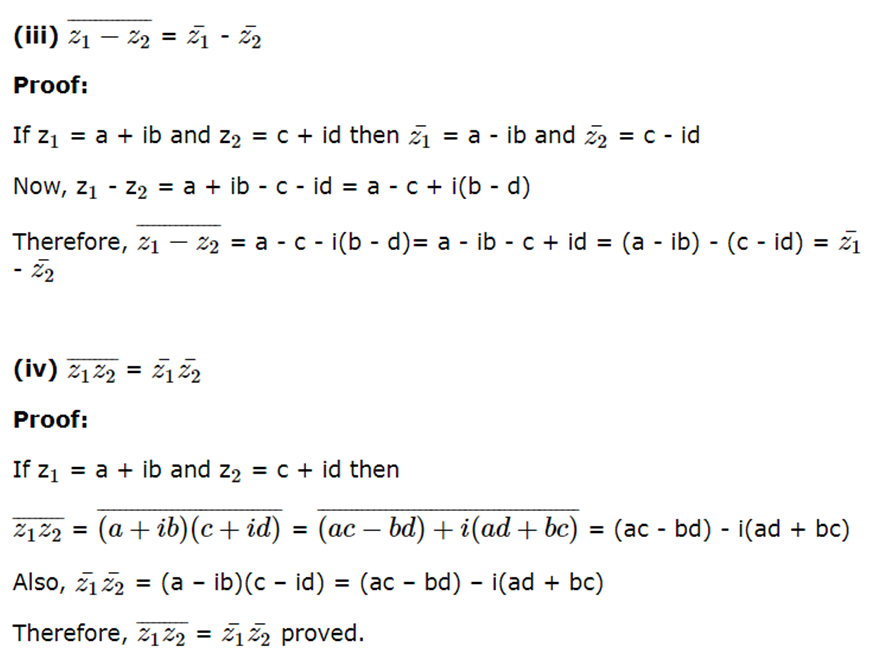



Modulus of Complex Number
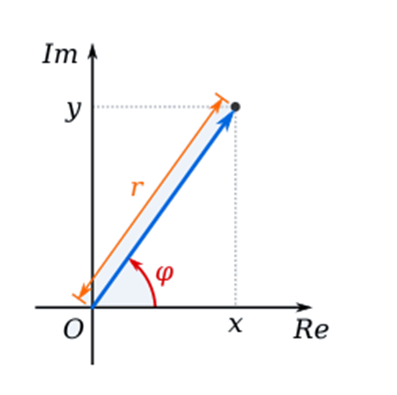
Modulus of the complex number is the distance of the point on the argand plane representing the complex number z from the origin.
Let P is the point that denotes the complex number z = x + iy.
Then OP = |z| = r = √(x2 + y2 ).
Properties of Modulus of Complex Number:

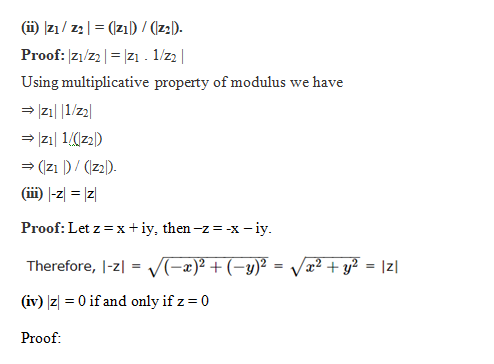
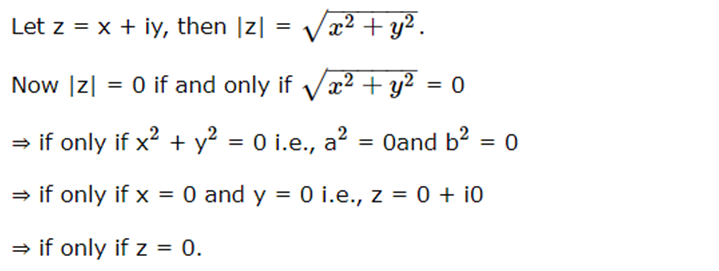
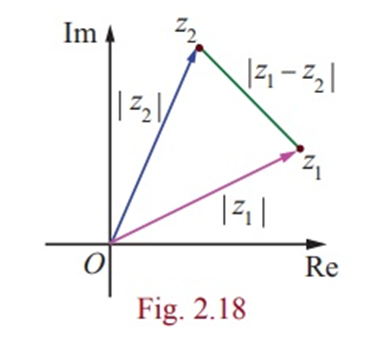
(v) For any two complex numbers z1 and z2, we have |z1 + z2| ≤ |z1| + |z2|.
Proof

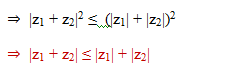
The distance between the two points z1 and z2 in complex plane is | z1 - z2 |
If we consider origin, z1 and z2 as vertices of a triangle, by the similar argument we have
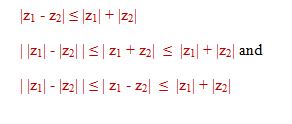
Geometrical interpretation
Now consider the triangle shown in figure with vertices O, z1 or z2 , and z1 + z2. We know from geometry that the length of the side of the triangle corresponding to the vector z1 + z2 cannot be greater than the sum of the lengths of the remaining two sides. This is the reason for calling the property as "Triangle Inequality".
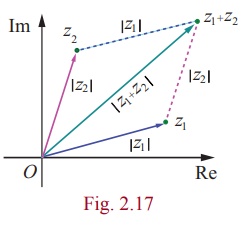
It can be generalized by means of mathematical induction to finite number of terms:
|z1 + z2 + z3 + …. + zn | ≤ |z1| + |z2| + |z3| + … + |zn| for n = 2,3,….
Note:
1. |z| > 0.
2. All the complex number with same modulus lie on the circle with centre origin and radius r = |z|.
Application:
Spectrum Analyzer

Those cool displays you see when music is playing? Yes, Complex Numbers are used to calculate them! Using something called "Fourier Transforms".
In fact many clever things can be done with sound using Complex Numbers, like filtering out sounds, hearing whispers in a crowd and so on.
It is part of a subject called "Signal Processing".
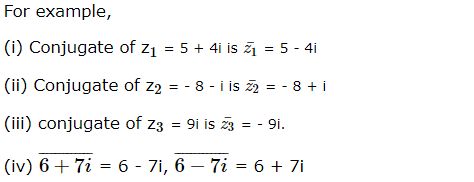
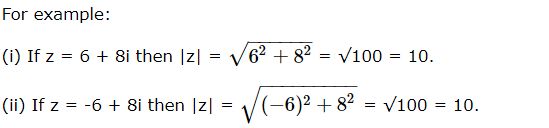
Example 1: Find the conjugate of the complex number z = (1 + 2i)/(1 – 2i).
Solution: z = (1 + 2i)/(1 – 2i)
Rationalising given complex number we have
⇒ z = ((1 + 2i)/(1 – 2i) )× (1 + 2i)/(1 + 2i)
⇒ z = (1 + 2i)2/(12 – (2i)2)
⇒ z = (1 + 4i2 + 4i)/(1 + 4)
⇒ z = (1 – 4 + 4i)/(1 + 4)
⇒ z = (-3 + 4i)/5
⇒![]() = (- 3 – 4i)/5.
= (- 3 – 4i)/5.
Example 2: Find the modulus of the complex number z = (3 – 2i)/2i
Solution: z = (3 – 2i)/2i
⇒ z = (3 )/2i – 2i/2i
⇒ z = 3/2i – 1
⇒ z = 3i/(2i2 ) – 1
⇒ z = (-3i/2) – 1
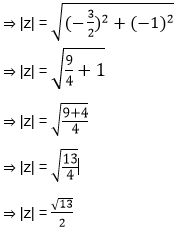
Example 3: If z + |z| = 1 + 4i, then find the value of |z|.
Solution: Let z = x + iy
⇒ z + |z| = 1 + 4i
⇒ x + iy + |x + iy| = 1 + 4i
⇒ x + iy + √(x2 + y2 ) = 1 + 4i
⇒ y = 4 and x + √(x2 + y2 ) = 1
⇒ x + √(x2 + 42 ) = 1
⇒ √(x2 + 42 ) = 1 – x
Squaring both side we have
⇒ x2 + 42 = 1 + x2 – 2x
⇒ 2x = -15
or x = -15/2
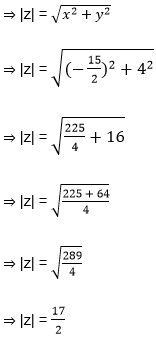
Argand Plane and Polar Representation
The plane having a complex number assigned to each of its point is called the
complex plane or the Argand plane.
Argument of Complex Numbers
The argument of a complex number is defined as the angle inclined from the real axis in the direction of the complex number represented on the complex plane. It is denoted by “θ” or “φ”. It is measured in the standard unit called “radians”.
The real and complex components of coordinates are found in terms of r and θ where r is the length of the vector, and θ is the angle made with the real axis.
The point P is uniquely determined by the ordered pair of real numbers (r, ), called the polar
coordinates of the point P.
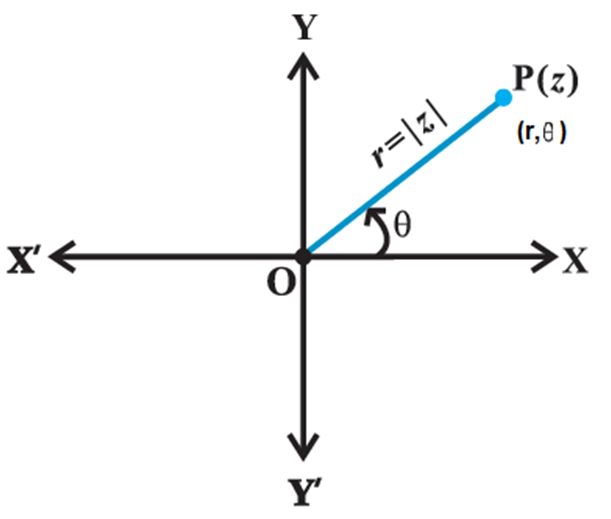
Polar Form of Complex Numbers
The argument of a complex number is defined as the angle inclined from the real axis in the direction of the complex number represented on the complex plane. It is denoted by “θ” or “φ”. It is measured in the standard unit called “radians”.
The real and complex components of coordinates are found in terms of r and θ where r is the length of the vector, and θ is the angle made with the real axis.
We have, x = r cos q, y = r sin q and therefore, z = r (cos q + i sin q).
The latter is said to be the polar form of the complex number.
q is called the argument (or amplitude) of z which is denoted by arg z. and
r = |z| =√(x2 + y2) is the modulus of z
For any complex number z ¹ 0, there corresponds only one value of q in 0 £ q < 2p.
The value of q such that – p < q £ p, called principal argument of z and is denoted by arg z.
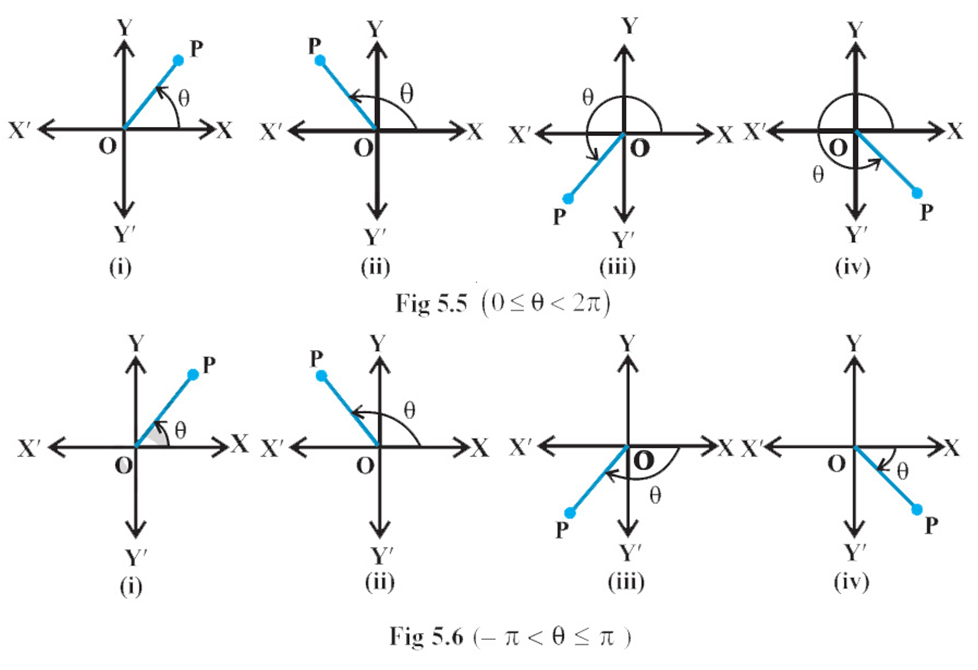

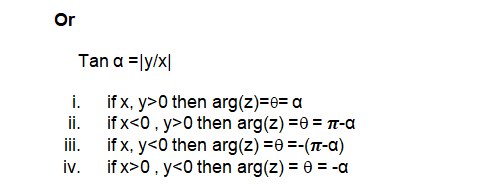
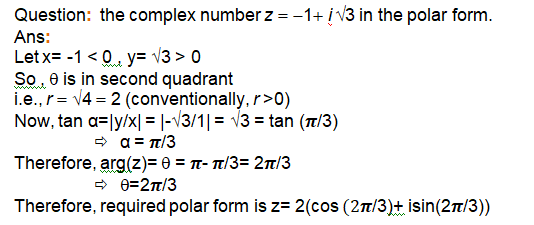
Properties of Argument of Complex Numbers
Suppose that z be a nonzero complex number and n be some integer, then
![]()
Let us assume, z1 and z2 be the two complex numbers, the following identities are:
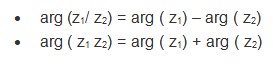
4. Solving Quadratic equations in complex numbers
- Books Name
- AMARENDRA PATTANAYAK Mathmatics Book
- Publication
- KRISHNA PUBLICATIONS
- Course
- CBSE Class 11
- Subject
- Mathmatics
Solving Quadratic equations in complex numbers
We can solve quadratic equations with complex coefficients using the quadratic formula. If the discriminant is zero, the equation has one repeated root. If all the coefficients are real, the root will be real. A general quadratic with complex coefficients can have any combination of real and nonreal roots.
The Quadratic Formula is ![]() The expression under the radical,
The expression under the radical, ![]() is called the discriminant. You can use the discriminant to determine the number and type of solutions an equation has.
is called the discriminant. You can use the discriminant to determine the number and type of solutions an equation has.
Using the discriminant, we identify the three different cases of quadratic equations as follows.
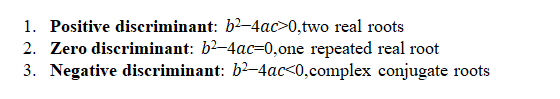
Solving Equations with Different Types of Solutions
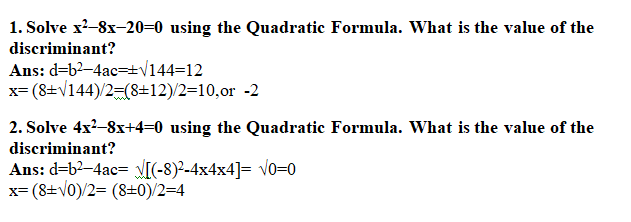

4. Nonreal Quadratic Equations with Real Negative Discriminants
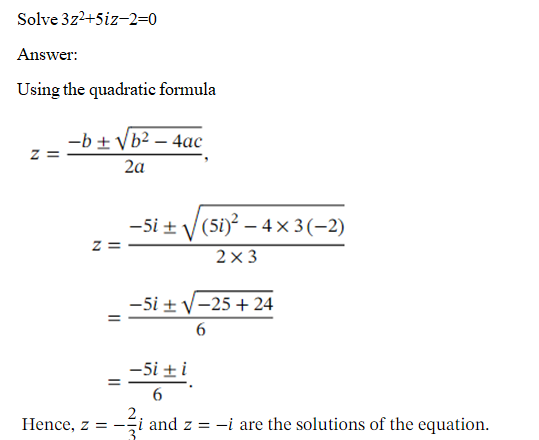
5. Square Root and Cube Root Of Complex Numbers
- Books Name
- AMARENDRA PATTANAYAK Mathmatics Book
- Publication
- KRISHNA PUBLICATIONS
- Course
- CBSE Class 11
- Subject
- Mathmatics
Square Root and Cube Root Of Complex Numbers:
Square roots of a complex number
Let the square root of a + ib be x + iy
That is = √[a+ib] = x + iy where x, y ∈ R
a + ib = ( x + iy )2 = x2 - y2 + i2xy
Equating real and imaginary parts, we get
x2 - y2 = a and 2xy = b
(x2 + y2 )2 = (x2 - y2 ) 2 + 4x2 y2 = a2 + b2
x2 + y2 = √[a2+b2], since x2 + y2 is positive
Solving x2 + y2 = a and x2+ y2 =√[a2+b2], we get
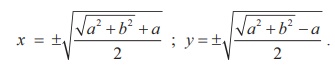
Since 2xy = b it is clear that both x and y will have the same sign when b is positive, and x and y have different signs when b is negative.
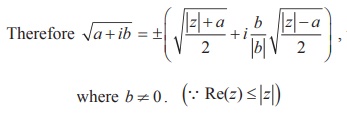
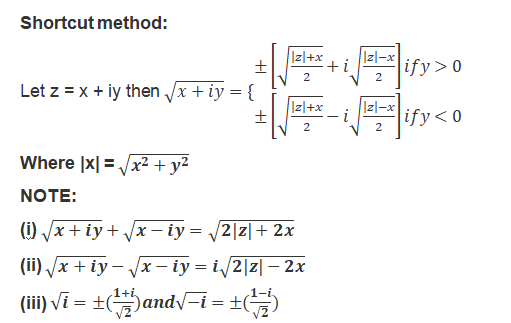
Formula for finding square root of a complex number
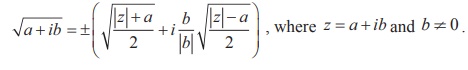
Note
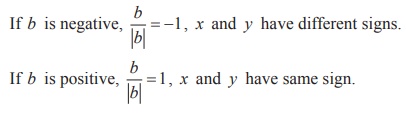
Example 1.
Find the square root of 6 - 8i.
Solution
We compute |6 - 8i| = √[62 + (-8)2] = 10
and applying the formula for square root, we get
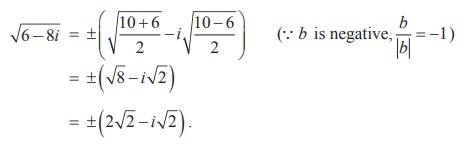
Example 2: Determine the square root of complex number 3 + 4i.
Solution: To determine the square root of 3 + 4i, we will determine its magnitude and compare with a + ib.
|3 + 4i| = √(32 + 42) = √(9 + 16) = √25 = 5; a = 3 and b = 4 > 0
Therefore, using formula for square root of complex numbers, we have
√(3 + 4i) = ± (√[√(5 + 3)/2] + i√[√(5 - 3)/2] )
= ± (√(8/2) + i √(2/2))
= ±(√4 + i√1)
= ± (2 + i)
Answer: Hence, √(3 + 4i) = ± (2 + i)
Cube Root of Unity Values:
Suppose the cube root of 1 is “x”,
i .e ., 3√1 = x.
According to the general cube roots definition,
x3 = 1
-
- x3 – 1 = 0
since (a3 – b3) = (a – b) ( a2 + ab + b2)
Now, (x3 – 13) = 0
-
- (x – 1)( x2 + x + 1) = 0
Therefore,
x = 1 Or ( x2 + x + 1) = 0
-
- x=1 or x = [(-1) ± √(12-4.1.1)]/2
- x=1 or x= [-1 ± √-3]/2
- x=1 or x= -1/2 ± i√(3)/2
Therefore, the three cube roots of unity are:
1, ω= -1/2+i√(3)/2, ω2= -1/2 – i√(3)/2
Properties of Cube roots of unity
1) One imaginary cube roots of unity is the square of the other imaginary cube root.
= ¼ [(-1)2 – 2 × 1 × √3 i + ( √3 i)2] = ¼(1 – 2√ 3i – 3) = (-1-√ 3 i) /2
And
= ¼ [(-1)2 + 2 × 1 × √3 i + ( √3 i)2] = ¼(1 + 2√ 3i – 3) = (-1+ √ 3 i) /2
i.e., ω2= (ω2)2
2) If two imaginary cube roots are multiplied then the product we get is equal to 1.
Let’s take ω = (-1 + √3 i ) /2
ω2 = (-1 – √3 i ) /2
Now we will get the product of two imaginary cube roots as ω x ω2 = [(-1 + √3 i ) / 2]x [(-1 – √3 i ) /2]
Or ω3 = ¼[(-1)2 – (√3 i)2]
= ¼[( 1 – 3i2) = ¼ x 4 = 1.
3) As there are three cube roots of unity, their sum is zero, let’s see how
= 1 + [(-1 + √3 i ) /2] + [(-1 – √3 i ) /2]
Or
1 + ω + ω2 = 1 – ½ + (√3 i ) /2 – ½ – (√3 i ) /2
= 0
4) conjugate of One imaginary cube roots of unity is equal to the other imaginary cube root.
Euler’s form of a Complex Number
eiƟ = cos Ɵ + i sin Ɵ
This form makes the study of complex numbers and its properties simple. Any complex number can be expressed as
z = x + iy (Cartesian form)
= |z| [cos Ɵ + I sin Ɵ] (polar form)
= |z| eiƟ
De Moivre’s Theorem and its Applications
(a) De Moivre’s Theorem for integral index. If n is a integer, then (cos Ɵ + i sin Ɵ)n = cos (nƟ) + i sin (nƟ)
(b) De Moivre’s Theorem for rational index. If n is a rational number, then the value of or one of the values of
(cosƟ + isinƟ)n is cos (nƟ) + i sin (nƟ).
In fact, if n = p/q where p, q ϵ I, q > 0 and p,q have no factors in common, then (cos Ɵ + i sin Ɵ)n has q distinct values, one of which is cos (nƟ) + i sin (nƟ)
The nth Roots of Unity
By an nth root of unity we mean any complex number z which satisfies the equation zn = 1 (1)
Since an equation of degree n has n roots, there are n values of z which satisfy the equation (1). To obtain these n values of z, we write 1 = cos (2kπ) + I sin (2kπ)
Where k ϵ I and ![]()
[using the De Moivre’s Theorem]
Where k = 0, 1, 2, …., n -1.
Note
The values of (cos Ɵ + I sin Ɵ)p/q where p, q ϵ I, q ≠ 0, hcf (p,q) = 1 are given by
![]()
Where k = 0, 1, 2, ….., q -1.

 KRISHNA PUBLICATIONS
KRISHNA PUBLICATIONS
