- Books Name
- Rakhiedu Mathematics Book
- Publication
- Param Publication
- Course
- CBSE Class 10
- Subject
- Mathmatics
3.3 algebraic methods of solving a pair of linear equations
There are four methods for solving a pair of linear equations
(i) Substitution method
(ii) Elimination method
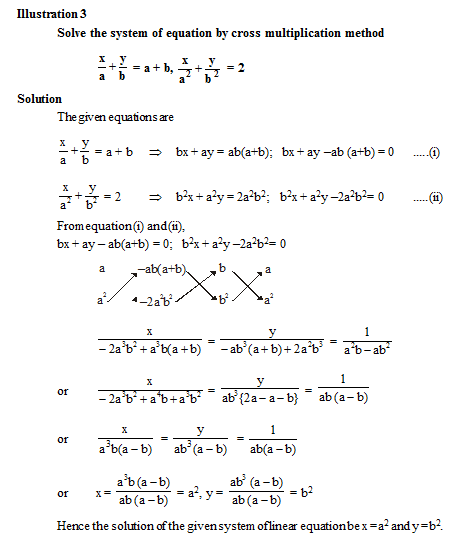
(iii) Cross-multiplication method
(iv) Graphical Method

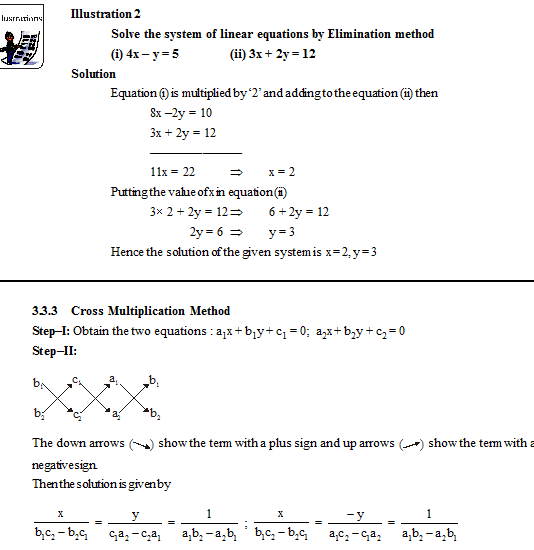
3.3.2 Elimination Method
Step–I: Obtain the two equations
Step–II: First multiply both the equations by some suitable non-zero constant to make the coefficient of one variable (either x or y) numerically equal.
Step–III: Add or subtract one equation from the other, then one variable gets eliminated.
Step–IV: Solve the equation in one variable.
Step–V: Substitute the value of x (or y) in any one of the given equations and find the value of another variable.
3.3.4 Graphical Method:
In graphical method, we draw the graph of both equations using same pair of horizontal and vertical lines called X-axis and Y-axis respectively. Coordinates of the point(s) of intersection of the two lines is/are the solution.
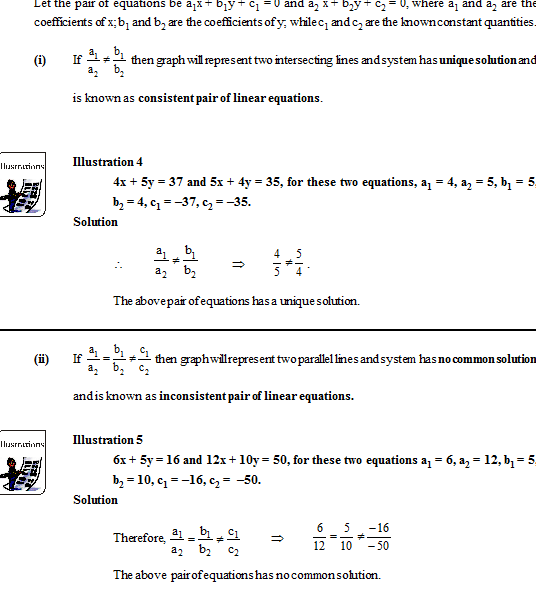
Nature of solutions:
When we try of solve a pair of equations we could arrive at three possible results. They are, having
(a) a unique solution
(b) an infinite number of solutions
(c) no solution

 Carrier Point
Carrier Point
