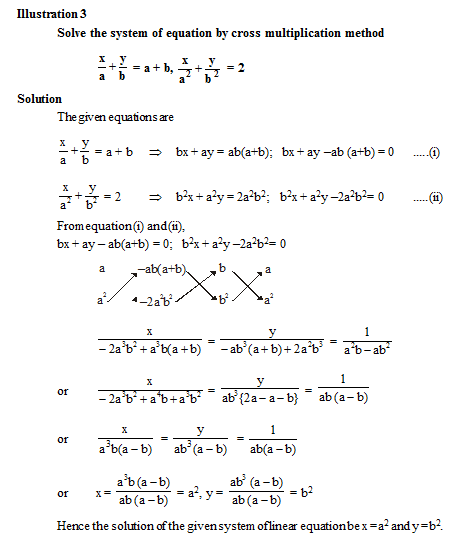
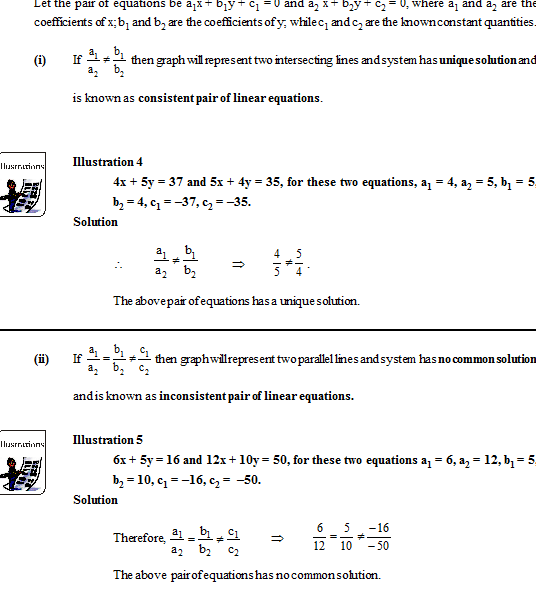
1. Solution of Two Linear Equations in Two Variables in Different Methods
- Books Name
- PragatiPublisher Mathematics Book
- Publication
- Pragati
- Course
- CBSE Class 10
- Subject
- Mathmatics

1. Solution of Two Linear Equations in Two Variables in Different Methods
- Books Name
- Mathematics Book for CBSE Class 10
- Publication
- Carrier Point
- Course
- CBSE Class 10
- Subject
- Mathmatics
Graphical Method of Solving Linear Equations In Two Variables
Let the system of pair of linear equations be
a1x + b1y = c1 ….(1)
a2x + b2y = c2 ….(2)
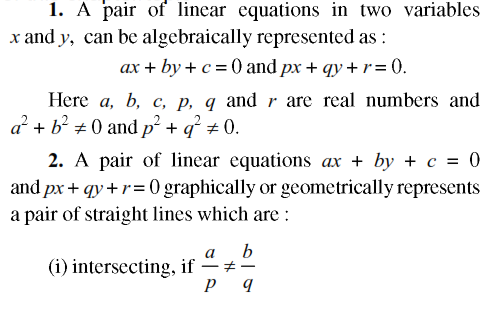
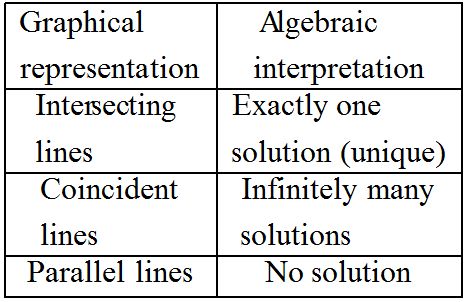
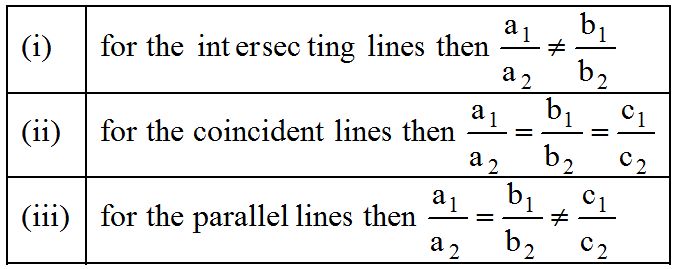
We know that given two lines in a plane, only one of the following three possibilities can happen –
(i) The two lines will intersect at one point.
(ii) The two lines will not intersect, however far they are extended, i.e., they are parallel.
(iii) The two lines are coincident lines.
Types Of Solutions:
There are three types of solutions
- Unique solution.
- Infinitely many solutions
- No solution.
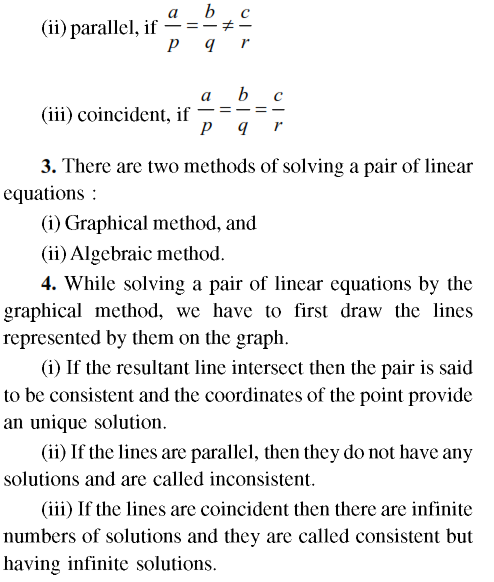
(A) Consistent: If a system of simultaneous linear equations has at least one solution then the system is said to be consistent.
(i) Consistent equations with unique solution: The graphs of two equations intersect at a unique point.
For Example Consider
x + 2y = 4
7x + 4y = 18
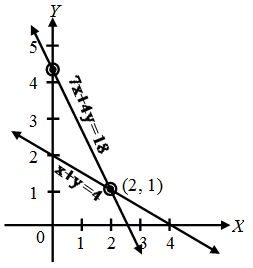
The graphs (lines) of these equations intersect each other at the point (2, 1) i.e., x = 2, y = 1.
Hence, the equations are consistent with unique solution.
(ii) Consistent equations with infinitely many solutions: The graphs (lines) of the two equations will be coincident.
For Example Consider 2x + 4y = 9 ⇒ 3x + 6y = 27/2
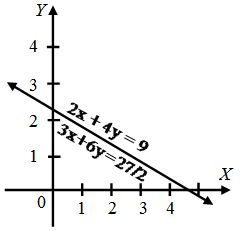
The graphs of the above equations coincide. Coordinates of every point on the lines are the solutions of the equations. Hence, the given equations are consistent with infinitely many solutions.
(B) Inconsistent Equation: If a system of simultaneous linear equations has no solution, then the system is said to be inconsistent.
No Solution: The graph (lines) of the two equations are parallel.
For Example Consider
4x + 2y = 10
6x + 3y = 6
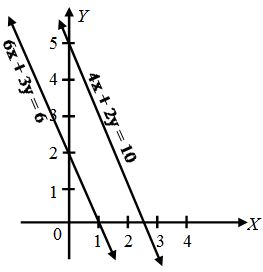
The graphs (lines) of the given equations are parallel. They will never meet at a point. So, there is no solution. Hence, the equations are inconsistent.

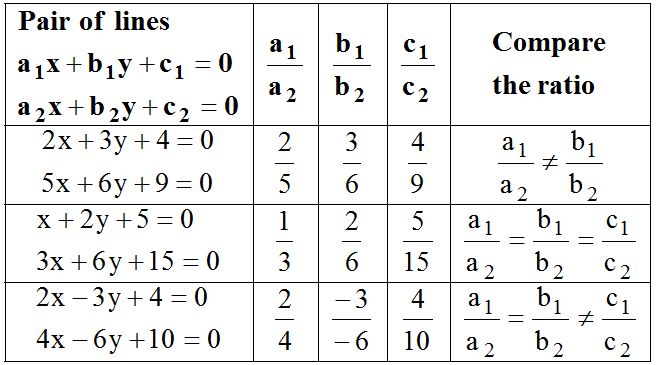
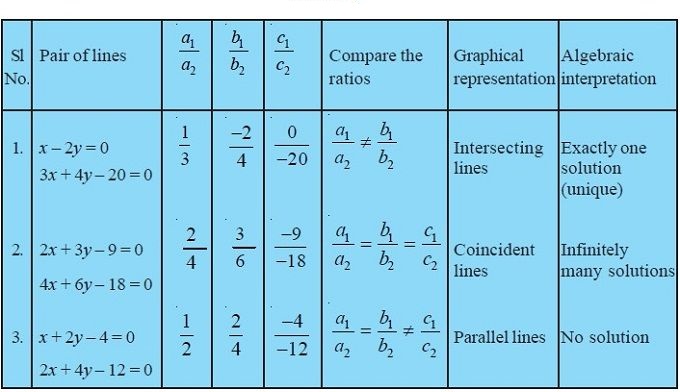
From the table above you can observe that if the line a1x + b1y + c1 = 0 and a2x + b2y + c2 = 0 are
Graphical Method Examples
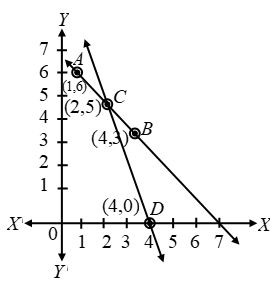
Example 1: The path of highway number 1 is given by the equation x + y = 7 and the highway number 2 is given by the equation 5x + 2y = 20. Represent these equations geometrically.
Sol. We have, x + y = 7
⇒ y = 7 – x ….(1)
In tabular form
X
1
4
Y
6
3
Points
A
B
and 5x + 2y = 20
⇒ y = 20−5x2 ….(2)
In tabular form
X
2
4
Y
5
0
Points
C
D
Plot the points A (1, 6), B(4, 3) and join them to form a line AB.
Similarly, plot the points C(2, 5). D (4, 0) and join them to get a line CD. Clearly, the two lines intersect at the point C. Now, every point on the line AB gives us a solution of equation (1). Every point on CD gives us a solution of equation (2).
Example 2: A father tells his daughter, “ Seven years ago, I was seven times as old as you were then. Also, three years from now, I shall be three times as old as you will be.” Represent this situation algebraically and graphically.
Sol. Let the present age of father be x years and that of daughter = y years
Seven years ago father’s age = (x – 7) years
Seven years ago daughter’s age = (y – 7) years
According to the problem
(x – 7) = 7(y – 7) or x – 7y = – 42 ….(1)
After 3 years father’s age = (x + 3) years
After 3 years daughter’s age = (y + 3) years
According to the condition given in the question
x + 3 = 3(y + 3) or x – 3y = 6 ….(2)
x – 7y = –42 ⇒ y=x+427
x
0
7
14
y
6
7
8
Points
A
B
C
x – 3y = 6 ⇒ y=x−63
x
6
12
18
y
0
2
4
Points
D
E
F
Plot the points A(0, 6), B(7, 7), C(14, 8) and join them to get a straight line ABC. Similarly plot the points D(6, 0), E(12, 2) and F(18, 4) and join them to get a straight line DEF.
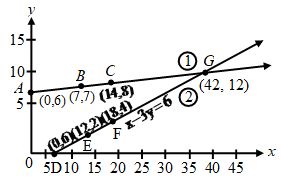
Example 3: 10 students of class X took part in a Mathematics quiz. If the number of girls is 4 more than the number of boys, find the number of boys and girls who took part in the quiz.
Sol. Let the number of boys be x and the number of girls be y.
Then the equations formed are
x + y = 10 ….(1)
and y = x + 4 ….(2)
Let us draw the graphs of equations (1) and (2) by finding two solutions for each of the equations. The
solutions of the equations are given.
x + y = 10 ⇒ y = 10 – x
X
0
8
Y
10
2
Points
A
B
y = x + 4
x
0
1
3
y
4
5
7
Points
C
D
E
Plotting these points we draw the lines AB and CE passing through them to represent the equations. The two lines AB and Ce intersect at the point E (3, 7). So, x = 3 and y = 7 is the required solution of the pair of linear equations.
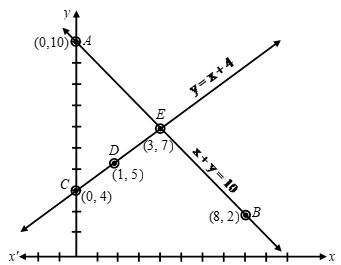
i.e. Number of boys = 3
Number of girls = 7.
Verification:
Putting x = 3 and y = 7 in (1), we get
L.H.S. = 3 + 7 = 10 = R.H.S., (1) is verified.
Putting x = 3 and y = 7 in (2), we get
7 = 3 + 4 = 7, (2) is verified.
Hence, both the equations are satisfied.
Example 4: Half the perimeter of a garden, whose length is 4 more than its width is 36m. Find the dimensions of the garden.
Sol. Let the length of the garden be x and width of the garden be y.
Then the equation formed are
x = y + 4 ….(1)
Half perimeter = 36
x + y = 36 ….(2)
y = x – 4
X
0
4
Y
-4
0
Points
A
B
y = 36 – x
X
10
20
Y
26
16
Points
C
D
Plotting these points we draw the lines AB and CD passing through them to represent the equations.
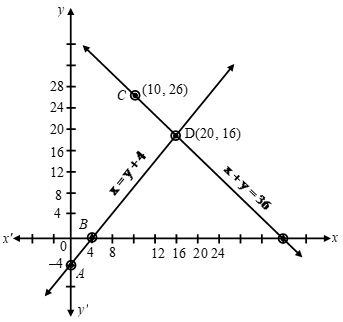
The two lines AB and CD intersect at the point (20, 16), So, x = 20 and y = 16 is the required solution of the pair of linear equations i.e. length of the garden is 20 m and width of the garden is 16 m.
Verification:
Putting x = 20 and y = 16 in (1). We get
20 = 16 + 4 = 20, (1) is verified.
Putting x = 20 and y = 16 in (2). we get
20 + 16 = 36
36 = 36, (2) is verified.
Hence, both the equations are satisfied.
Example 5: Draw the graphs of the equations x – y + 1 = 0 and 3x + 2y – 12 = 0. Determine the coordinates of the vertices of the triangle formed by these lines and the x-axis, and shade the triangular region.
Sol. Pair of linear equations are:
x – y + 1 = 0 ….(1)
3x + 2y – 12 = 0 ….(2)
x – y + 1 = 0 ⇒ y = x + 1
x
0
4
y
1
5
Points
A
B
3x + 2y – 12 = 0 ⇒ y = 12−3x2
x
0
2
y
6
3
Points
C
D
Plot the points A(0, 1), B(4, 5) and join them to get a line AB. Similarly, plot the points C(0, 6), D(2, 3) and join them to form a line CD.

Clearly, the two lines intersect each other at the point D(2, 3). Hence x = 2 and y = 3 is the solution of the
given pair of equations.
The line CD cuts the x-axis at the point
E (4, 0) and the line AB cuts the x-axis at the point F(–1, 0).
Hence, the coordinates of the vertices of the triangle are; D(2, 3), E(4, 0), F(–1, 0).
Verification:
Both the equations (1) and (2) are satisfied by x = 2 and y = 3. Hence, Verified.
Example 6: Show graphically that the system of equations
x – 4y + 14 = 0; 3x + 2y – 14 = 0 is consistent with unique solution.
Sol. The given system of equations is
x – 4y + 14 = 0 ….(1)
3x + 2y – 14 = 0 ….(2)
x – 4y + 14 = 0 ⇒ y = x+144
x
6
-2
y
5
3
Points
A
B
3x + 2y – 14 = 0 ⇒ y = −3x+142
x
0
4
y
7
1
Points
C
D

The given equations representing two lines, intersect each other at a unique point (2, 4). Hence, the equations are consistent with unique solution.
Example 7: Show graphically that the system of equations
2x + 5y = 16; 3x+152=24 has infinitely many solutions.
Sol. The given system of equations is
2x + 5y = 16 ….(1)
3x+152=24 ….(2)
2x + 5y = 16 ⇒ y = 16−2x5
x
-2
3
y
4
2
Points
A
B
3x+152=24 ⇒ y = 48−6x15
x
1/2
11/2
y
3
1
Points
C
D

The lines of two equations are coincident. Coordinates of every point on this line are the solution.
Hence, the given equations are consistent with infinitely many solutions.
Example 8: Show graphically that the system of equations
2x + 3y = 10, 4x + 6y = 12 has no solution.
Sol. The given equations are
2x + 3y = 10 ⇒ y = 10−2x3
x
-4
2
y
6
2
Points
A
B
4x + 6y = 12 ⇒ y = 12−4x6
x
-3
3
y
4
0
Points
C
D
Plot the points A (–4, 6), B(2, 2) and join them to form a line AB. Similarly, plot the points C(–3, 4), D(3, 0) and join them to get a line CD.
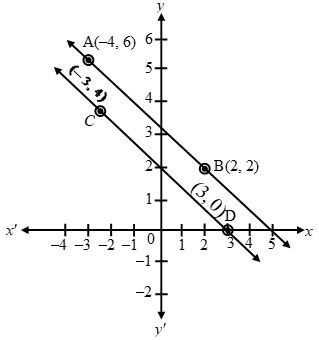
Clearly, the graphs of the given equations are parallel lines. As they have no common point, there is no common solution. Hence, the given system of equations has no solution.
Example 9: Given the linear equation 2x + 3y – 8 = 0, write another linear equation in two variables such that the
geometrical representing of the pair so formed is :
(i) intersecting lines
(ii) parallel lines
(iii) coincident lines
Sol. We have, 2x + 3y – 8 = 0
(i) Another linear equation in two variables such that the geometrical representation of the pair so formed is intersecting lines is
3x – 2y – 8 = 0
(ii) Another parallel lines to above line is
4x + 6y – 22 = 0
(iii) Another coincident line to above line is
6x + 9y – 24 = 0
Example 10: Solve the following system of linear equations graphically;
3x + y – 11 = 0 ; x – y – 1 = 0
Shade the region bounded by these lines and also y-axis. Then, determine the areas of the region bounded by these lines and y-axis.
Sol. We have,
3x + y – 11 = 0 and x – y – 1 = 0
(a) Graph of the equation 3x + y – 11 = 0
We have, 3x + y – 11 = 0
⇒ y = – 3x + 11
When, x = 2, y = –3 × 2 + 11 = 5
When, x = 3, y = – 3 × 3 + 11 = 2
Plotting the points P (2, 5) and Q(3, 2) on the graph paper and drawing a line joining between them, we get the graph of the equation 3x + y – 11 = 0 as shown in fig.
(b) Graph of the equation x – y – 1 = 0
We have,
x – y – 1 = 0
y = x – 1
When, x = – 1, y = –2
When, x = 3, y = 2
Plotting the points R(–1, –2) and S(3, 2) on the same graph paper and drawing a line joining between them, we get the graph of the equation x – y – 1 = 0 as shown in fig.

You can observe that two lines intersect at
Q(3, 2). So, x = 3 and y = 2. The area enclosed by the lines represented by the given equations and also the y-axis is shaded.
So, the enclosed area = Area of the shaded portion
= Area of ∆QUT = 1/2 × base × height
= 1/2 × (TU × VQ) = 1/2 × (TO + OU) × VQ
= 1/2 (11 + 1) 3 = 1/2 × 12 × 3 = 18 sq.units.
Hence, required area is 18 sq. units.
Example 11: Draw the graphs of the following equations
2x – 3y = – 6; 2x + 3y = 18; y = 2
Find the vertices of the triangles formed and also find the area of the triangle.
Sol. (a) Graph of the equation 2x – 3y = – 6;
We have, 2x – 3y = – 6 ⇒ y = 2x+63
When, x = 0, y = 2
When, x = 3, y = 4
Plotting the points P(0, 2) and Q(3, 4) on the graph paper and drawing a line joining between them we get the graph of the equation 2x – 3y = – 6 as shown in fig.
(b) Graph of the equation 2x + 3y = 18;
We have 2x + 3y = 18 ⇒ y = −2x+183
When, x = 0, y = 6
When, x = – 3, y = 8
Plotting the points R(0, 6) and S(–3, 8) on the same graph paper and drawing a line joining between them, we get the graph of the equation 2x + 3y = 18 as shown in fig.
(c) Graph of the equation y = 2
It is a clear fact that y = 2 is for every value of x. We may take the points T (3, 2), U(6, 2) or any other values.
Plotting the points T(3, 2) and U(6, 2) on the same graph paper and drawing a line joining between them, we get the graph of the equation y = 2 as shown in fig.
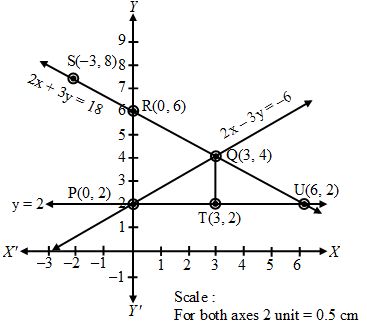
From the fig., we can observe that the lines taken in pairs intersect each other at points Q(3, 4), U (6, 2) and P(0, 2). These form the three vertices of the triangle PQU.
To find area of the triangle so formed
The triangle is so formed is PQU (see fig.)
In the ∆PQU
QT (altitude) = 2 units
and PU (base) = 6 units
so, area of ∆PQU = (base × height)
= 1/2 (PU × QT) = 1/2 × 6 × 2 sq. untis
= 6 sq. units.
Example 12: On comparing the ratios a1a2,b1b2andc1c2 and without drawing them, find out whether the lines representing the following pairs of linear equations intersect at a point, are parallel or coincide.
(i) 5x – 4y + 8 = 0, 7x + 6y – 9 = 0
(ii) 9x + 3y + 12 = 0, 18x + 6y + 24 = 0
(iii) 6x – 3y + 10 = 0, 2x – y + 9 = 0
Sol. Comparing the given equations with standard forms of equations a1x + b1y + c1 = 0 and
a2x + b2y + c2 = 0 we have,
(i) a1 = 5, b1 = – 4, c1 = 8;
a2 = 7, b2 = 6, c2 = – 9
a1a2=57,b1b2=−46
⇒a1a2≠b1b2
Thus, the lines representing the pair of linear equations are intersecting.
(ii) a1 = 9, b1 = 3, c1 = 12;
a2 = 18, b2 = 6, c2 = 24
a1a2=918=12,b1b2=36=12andc1c2=1224=12
⇒a1a2=b1b2=c1c2
Thus, the lines representing the pair of linear equation coincide.
(iii) a1 = 6, b1 = – 3, c1 = 10;
a2 = 2, b2 = – 6, c2 = 9
a1a2=62=3,b1b2=−3−1=3andc1c2=109
⇒a1a2=b1b2≠c1c2
Thus, the lines representing the pair of linear equations are parallel.
1. Solution of Two Linear Equations in Two Variables in Different Methods
- Books Name
- Rakhiedu Mathematics Book
- Publication
- Param Publication
- Course
- CBSE Class 10
- Subject
- Mathmatics
3.1 INTRODUCTION
While solving the problems, in most cases, first we need to frame an equation. In this chapter, we learn how to frame and solve equations. There are given some methods to solve these equations. We will further study about word problems and application of simultaneous equations.
Equation: A statement in which two algebraic expressions are equal is known as equation.
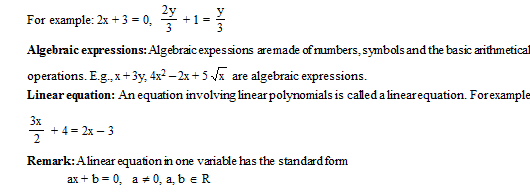
Solution (root) of a linear equation: The value of the variable which makes the two sides of the equation equal and satisfies the equation is called the solution of the equation.
Rules for solving an equation:
(i) The same number is added or subtracted to both sides of an equation, the resulting equation is equivalent to the first.
(ii) If both sides of an equation are multiplied by the same non-zero number the resulting equation is equivalent to the first.
Remark: Every linear equation in one variable has only one (unique) solution.
In this chapter we shall study about system of linear equation in two variables, solution of a system of linear equations in two variables.
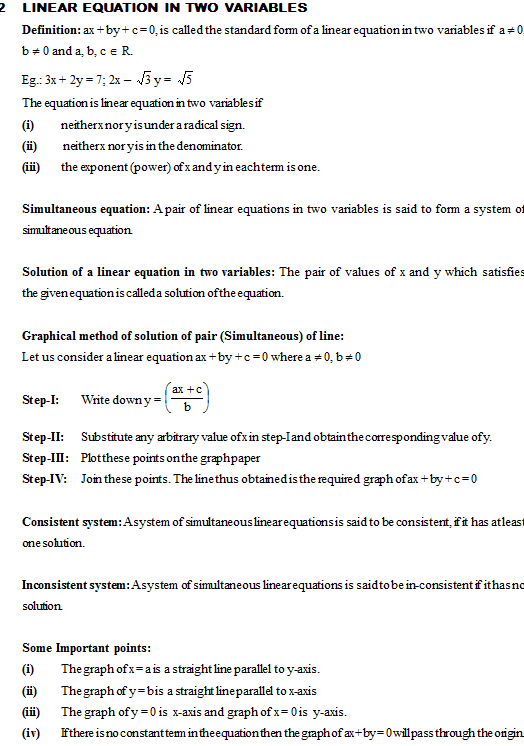
3.3 algebraic methods of solving a pair of linear equations
There are four methods for solving a pair of linear equations
(i) Substitution method
(ii) Elimination method
(iii) Cross-multiplication method
(iv) Graphical Method
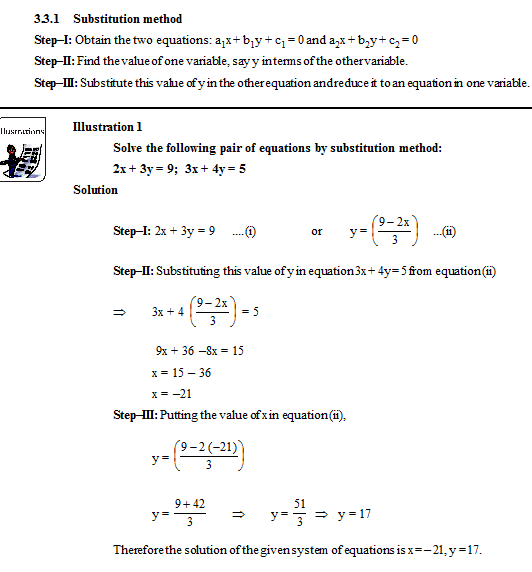
3.3.2 Elimination Method
Step–I: Obtain the two equations
Step–II: First multiply both the equations by some suitable non-zero constant to make the coefficient of one variable (either x or y) numerically equal.
Step–III: Add or subtract one equation from the other, then one variable gets eliminated.
Step–IV: Solve the equation in one variable.
Step–V: Substitute the value of x (or y) in any one of the given equations and find the value of another variable.
Geometric representation of different possibilities of solutions/inconsistency?
- Books Name
- Mathematics Book for CBSE Class 10
- Publication
- Carrier Point
- Course
- CBSE Class 10
- Subject
- Mathmatics
Basics Revisited
Equation
An equation is a statement that two mathematical expressions having one or more variables are equal.
Linear Equation
Equations in which the powers of all the variables involved are one are called linear equations. The degree of a linear equation is always one.
General form of a Linear Equation in Two Variables
The general form of a linear equation in two variables is ax + by + c = 0, where a and b cannot be zero simultaneously.
Representing linear equations for a word problem
To represent a word problem as a linear equation
- Identify unknown quantities and denote them by variables.
- Represent the relationships between quantities in a mathematical form, replacing the unknowns with variables.
Solution of a Linear Equation in 2 variables
The solution of a linear equation in two variables is a pair of values, one for x and the other for y, which makes the two sides of the equation equal.
Eg: If 2x+y=4, then (0,4) is one of its solutions as it satisfies the equation. A linear equation in two variables has infinitely many solutions.
Geometrical Representation of a Linear Equation
Geometrically, a linear equation in two variables can be represented as a straight line.
2x – y + 1 = 0
⇒ y = 2x + 1

Graph of y = 2
x
+1
Plotting a Straight Line
The graph of a linear equation in two variables is a straight line. We plot the straight line as follows:

Any additional points plotted in this manner will lie on the same line.
All about Lines
General form of a pair of linear equations in 2 variables
A pair of linear equations in two variables can be represented as follows

The coefficients of x and y cannot be zero simultaneously for an equation.
Nature of 2 straight lines in a plane
For a pair of straight lines on a plane, there are three possibilities
i) They intersect at exactly one point

pair of linear equations which intersect at a single point.
ii) They are parallel

pair of linear equations which are parallel.
iii) They are coincident

pair of linear equations which are coincident.
Graphical Solution
Representing pair of LE in 2 variables graphically
Graphically, a pair of linear equations in two variables can be represented by a pair of straight lines.
Graphical method of finding solution of a pair of Linear Equations
Graphical Method of finding the solution to a pair of linear equations is as follows:
- Plot both the equations (two straight lines)
- Find the point of intersection of the lines.
The point of intersection is the solution.
Comparing the ratios of coefficients of a Linear Equation

Algebraic Solution
Finding solution for consistent pair of Linear Equations
The solution of a pair of linear equations is of the form (x,y) which satisfies both the equations simultaneously. Solution for a consistent pair of linear equations can be found out using
i) Elimination method
ii) Substitution Method
iii) Cross-multiplication method
iv) Graphical method
Substitution Method of finding solution of a pair of Linear Equations
Substitution method:
y – 2x = 1
x + 2y = 12
(i) express one variable in terms of the other using one of the equations. In this case, y = 2x + 1.
(ii) substitute for this variable (y) in the second equation to get a linear equation in one variable, x. x + 2 × (2x + 1) = 12
⇒ 5 x + 2 = 12
(iii) Solve the linear equation in one variable to find the value of that variable.
5 x + 2 = 12
⇒ x = 2
(iv) Substitute this value in one of the equations to get the value of the other variable.
y = 2 × 2 + 1
⇒y = 5
So, (2,5) is the required solution of the pair of linear equations y – 2x = 1 and x + 2y = 12.
Elimination method of finding solution of a pair of Linear Equations
Elimination method
Consider x + 2y = 8 and 2x – 3y = 2
Step 1: Make the coefficients of any variable the same by multiplying the equations with constants. Multiplying the first equation by 2, we get,
2x + 4y = 16
Step 2: Add or subtract the equations to eliminate one variable, giving a single variable equation.
Subtract second equation from the previous equation
2x + 4y = 16
2x – 3y = 2
– + –
———————–
0(x) + 7y =14
Step 3: Solve for one variable and substitute this in any equation to get the other variable.
y = 2,
x = 8 – 2 y
⇒ x = 8 – 4
⇒ x = 4
(4, 2) is the solution.
Geometric representation of different possibilities of solutions/inconsistency?
- Books Name
- Rakhiedu Mathematics Book
- Publication
- Param Publication
- Course
- CBSE Class 10
- Subject
- Mathmatics
3.3 algebraic methods of solving a pair of linear equations
There are four methods for solving a pair of linear equations
(i) Substitution method
(ii) Elimination method
(iii) Cross-multiplication method
(iv) Graphical Method

3.3.2 Elimination Method
Step–I: Obtain the two equations
Step–II: First multiply both the equations by some suitable non-zero constant to make the coefficient of one variable (either x or y) numerically equal.
Step–III: Add or subtract one equation from the other, then one variable gets eliminated.
Step–IV: Solve the equation in one variable.
Step–V: Substitute the value of x (or y) in any one of the given equations and find the value of another variable.
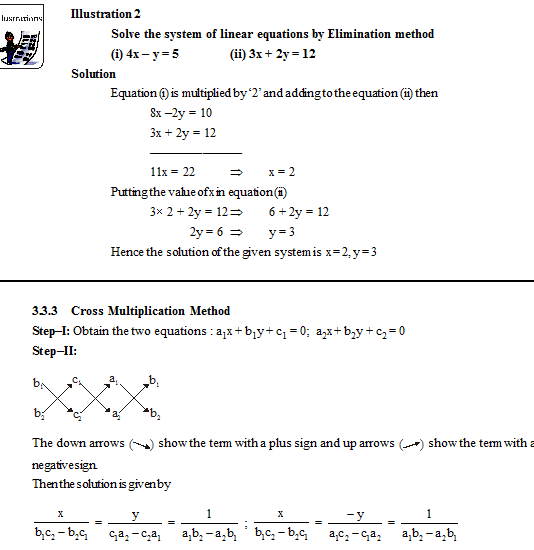
3.3.4 Graphical Method:
In graphical method, we draw the graph of both equations using same pair of horizontal and vertical lines called X-axis and Y-axis respectively. Coordinates of the point(s) of intersection of the two lines is/are the solution.
Nature of solutions:
When we try of solve a pair of equations we could arrive at three possible results. They are, having
(a) a unique solution
(b) an infinite number of solutions
(c) no solution
Algebraic conditions for number of solutions?
- Books Name
- Rakhiedu Mathematics Book
- Publication
- Param Publication
- Course
- CBSE Class 10
- Subject
- Mathmatics
3.3 algebraic methods of solving a pair of linear equations
There are four methods for solving a pair of linear equations
(i) Substitution method
(ii) Elimination method
(iii) Cross-multiplication method
(iv) Graphical Method

3.3.2 Elimination Method
Step–I: Obtain the two equations
Step–II: First multiply both the equations by some suitable non-zero constant to make the coefficient of one variable (either x or y) numerically equal.
Step–III: Add or subtract one equation from the other, then one variable gets eliminated.
Step–IV: Solve the equation in one variable.
Step–V: Substitute the value of x (or y) in any one of the given equations and find the value of another variable.


3.3.4 Graphical Method:
In graphical method, we draw the graph of both equations using same pair of horizontal and vertical lines called X-axis and Y-axis respectively. Coordinates of the point(s) of intersection of the two lines is/are the solution.
Nature of solutions:
When we try of solve a pair of equations we could arrive at three possible results. They are, having
(a) a unique solution
(b) an infinite number of solutions
(c) no solution

Solution of a pair of linear equations in two variables algebraically - by substitution, by elimination and by cross multiplication method?
- Books Name
- Rakhiedu Mathematics Book
- Publication
- Param Publication
- Course
- CBSE Class 10
- Subject
- Mathmatics
3.6 Solution of a system of a pair of equations reducible to the system of a pair of linear equations in two variables
By using the suitable substitution or simplification first we convert the given system into the system of a pair of linear equations in two variables. Then after using any algebraic or graphical method we solve the system.
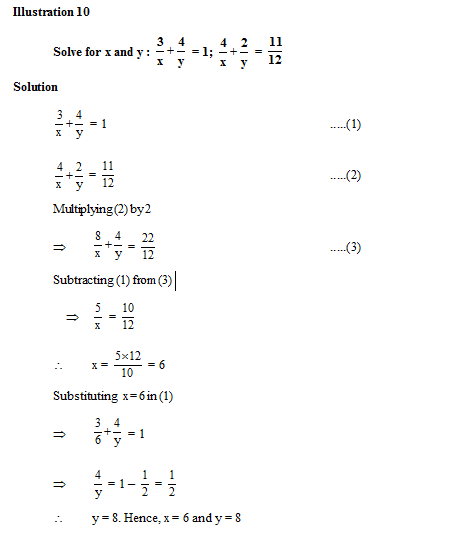

 Pragati
Pragati
